A Nice Polynomial Expression

🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts).
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
/ @sybermath
/ @aplusbi
⭐ Join this channel to get access to perks:→ bit.ly/3cBgfR1
My merch → teespring.com/...
Follow me → / sybermath
Subscribe → www.youtube.co...
⭐ Suggest → forms.gle/A5bG...
If you need to post a picture of your solution or idea:
in...
#algebra #polynomials #polynomial
via @RUclips @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
▶ Trigonometry: • Trigonometry
▶ Algebra: • Algebra
▶ Complex Numbers: • Complex Numbers
▶ Calculus: • Calculus
▶ Geometry: • Geometry
▶ Sequences And Series: • Sequences And Series

[@mystychief]

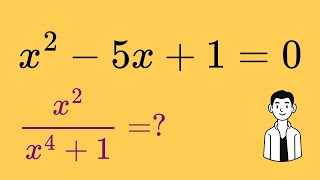
? = x(x^4-29) makes it easier. x = sqrt(2)+1. x² = 3+2sqrt(2). x^4 = 17+12sqrt(2). Fill in ? = (sqrt(2)+1)(17+12sqrt(2)-29) = (sqrt(2)+1)(-12+12sqrt(2)) = ... = 12.

[@ianfowler9340]

Or you could just use your method on x=√2+1. AKA The Silver Ratio!
x^2=3√2+1
x^3=5√2+7
x^5=29√2+41
x^5-29x=41-29=12
But keeping the x as a variable is a sweet solution.

[@nasrullahhusnan2289]

x⁵-29x=x(x⁴-29)
x=1+sqrt(2) --> x²=3+2sqrt(2)
x⁴=17+12sqrt(2)
x⁴-29=-12[1-sqrt(2)]
x(x⁴-29)=-12[1+sqrt(2)][1-sqrt(2)]
=-12[(-1)
=12
Common procedure:
x=1+sqrt(2)
x⁵=[1+sqrt(2)]⁵
=1+5sqrt(2)+10(2)+10[2sqrt(2)]
+5(20)+4sqrt(2)
=121+29sqrt(2)
29x=29+29sqrt(2)
x⁵-29x=92

[@paulortega5317]

Or a recursive approach...
x₁ y₁
x₂ = x₁ + 2y₁ y₂ = x₁ + y₁
Also x₃ = 2x₂ + x₁ and y₃ = 2y₂ + y₁
1 1
3 2
7 5
17 12
41 29
Plus the value pairs, alternately, are solutions for the diophantine equations x² = 2y² - 1 and x² = 2y² + 1

[@wes9627]

x^2=2x+1; x^3=2x^2+x=5x+2; x^5=(2x+1)(5x+2)=10x^2+9x+2=29x+12
x^5-29x=29x+12-29x=12

[@trojanleo123]

Answer = 12