p(p+y)=x(x+y)
HTML-код
- Опубликовано: 27 дек 2024
- Hey guys!
Here is the video of a problem from Differential Equations of First Order But Not of First Degree. We have solved the equation using solvable for p method.
My hearty thanks to all the subscribers, supporters, viewers and well-wishers❤.
With Love,
Chinnaiah Kalpana🍁
Note:
An equation of the form f(x,y,p)=0, where p is not of first degree, is called a differential equation of first order and not of first degree.
An equation of the form
p^n + P1(x,y) p^n-1 +P2(x,y) p^n-2 +...+ Pn-1(x,y) p + Pn(x,y) =0 is called the general first order equation of degree n(greater than 1).
These equations can be divided into four types:
(i)Solvable for p
(ii)Solvable for x
(iii)Solvable for y
(iv)Clairaut's Equation.
Equations Solvable for p:
Consider the first order and higher degree (say n) equation is in the form of p^n + A1 p^n-1 + A2 p^n-2 +...+ An=0.
Where A1 , A2 , ... , An are functions of x and y.
If it is solvable for p then rearrange the left hand side of the above equation into n linear factors.
[p-f1(x,y)][p-f2(x,y)]....[p-fn(x,y)]=0
Equating each linear factors to zero, we get
p-f1(x,y)=0, p-f2(x,y)=0 ,..., p-fn(x,y)=0
Each of these equations is of the first order and first degree and it can be solved by the any one of the known methods.
If the solutions of the above n component equations are
g1(x,y,c)=0, g2(x,y,c)=0, .... , gn(x,y,c)=0.
Then the general solution of the given differential equation is a combination of above solutions are given by the form
g1(x,y,c)g2(x,y,c)....gn(x,y,c)=0.
For more such videos👇
• Solvable for p
Stay tuned to 'Maths Pulse'.
Get rid of 'Maths Phobia'.
Have a happy learning!
#solvableforp #differentialequations #differentialequationsoffirstorderbutnotoffirstdegree #mathspulse #chinnaiahkalpana #solvableforpexamples #solvableforpproblems #equationssolvableforp #maths #math #engineeringmathematics #engineeringmaths #bscmathematics #bscmaths #mathematics


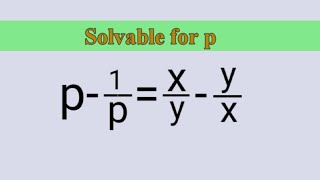




![Noob To Max With DRAGON REWORK In Blox Fruits [FULL MOVIE]](http://i.ytimg.com/vi/LnBMOinoOvA/mqdefault.jpg)
![Solve:p(p-y)=x(x+y). [ Diffential equations of the first order but not of the first degree ]](/img/1.gif)