3-3 Reduction, Physical Reductions

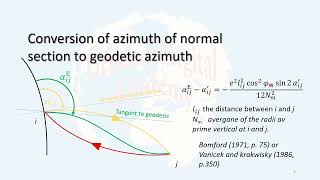
All measurements are conducted on the Earth's surface using planar geometry, where distances are defined as straight lines and angles as parts of a plane limited by those lines. In this case, the datum is a plane. However, the Earth's horizontal datum is an ellipsoid, which follows a different geometry. Distances on this ellipsoid are geodesic curves rather than straight lines, and angles are parts of the ellipsoidal surface rather than a plane. The process of converting measurements from planar geometry to their corresponding values on the ellipsoid's surface is called reduction.
Since all measurements occur within the Earth's gravity field, it is essential to remove the influence of gravity to make them purely geometric. When we level an instrument at the Earth's surface, we align its principal axis with the plumbline. However, for geodetic purposes, we need measurements relative to the ellipsoid's normal rather than the plumbline. The angle between the local vertical (plumbline) and the normal to the ellipsoid is known as the deflection of the vertical. This angle can be used to compute how much it affects horizontal and vertical directions, a process known as physical reduction.
In this video lecture, the principles of reduction, along with the geometric interpretation and the concept of physical reductions in both vertical and horizontal directions, are explained.