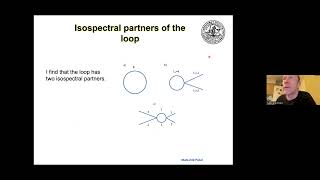
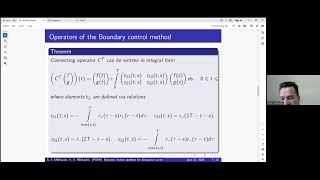
Vladimir Sivkin | Numerical implementation of multipoint formulas in inverse problems

Days on Diffraction 2024. Mini-symposium “Inverse Problems”. Wednesday, 12 June, 2024
Vladimir N. Sivkin (Lomonosov MSU),
Roman G. Novikov (Ecole polytechnique de Paris)
Numerical implementation of multipoint formulas in inverse problems
We present the first numerical study of multipoint formulas for finding leading coefficients in asymptotic expansions arising in scattering theory. In particular, we implement different formulas for finding the Fourier transform of potential from the scattering amplitude at several high energies. We show that the aforementioned approach can be used for essential numerical improvements of classical results including the slowly convergent Born-Faddeev formula for inverse scattering at high energies. The approach of multipoint formulas can be also used for recovering the X-ray transform of
potential from boundary values of the scattering wave functions at several high energies. In addition, we show that the aforementioned multipoint formulas admit an efficient regularization for the case of random noise. This talk is based on [1]. In particular, we proceed from theoretical works [2, 3].
References
[1] R. G. Novikov, V. N. Sivkin, G. V. Sabinin, Multipoint formulas in inverse problems and their numerical implementation, Inverse Problems, 39(12), 125016 (2023).
[2] R. G. Novikov, Multipoint formulas for scattered far field in multidimensions, Inverse Problems, 36, 095001 (2020).
[3] R. G. Novikov, Multipoint formulas for inverse scattering at high energies, Russ. Math. Surv., 76(4), 723-725 (2021).