An Interesting Olympiad Problem
HTML-код
- Опубликовано: 21 окт 2024
- 🤩 Hello everyone, I'm very excited to bring you a new channel (aplusbi)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
/ @sybermathshorts
/ @aplusbi
❤️ ❤️ ❤️ My Amazon Store: www.amazon.com...
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you.
If you are preparing for Math Competitions and Math Olympiads, then this is the page for you!
CHECK IT OUT!!! ❤️ ❤️ ❤️
❤️ A Differential Equation | The Result Will Surprise You! • A Differential Equatio...
❤️ Crux Mathematicorum: cms.math.ca/pu...
❤️ A Problem From ARML-NYSML Math Contests: • A Problem From ARML-NY...
❤️ LOGARITHMIC/RADICAL EQUATION: • LOGARITHMIC/RADICAL EQ...
❤️ Finding cos36·cos72 | A Nice Trick: • Finding cos36·cos72 | ...
⭐ Join this channel to get access to perks:→ bit.ly/3cBgfR1
My merch → teespring.com/...
Follow me → / sybermath
Subscribe → www.youtube.co...
⭐ Suggest → forms.gle/A5bG...
If you need to post a picture of your solution or idea:
in...
#radicals #radicalequations #algebra #calculus #differentialequations #polynomials #prealgebra #polynomialequations #numbertheory #diophantineequations #comparingnumbers #trigonometry #trigonometricequations #complexnumbers #math #mathcompetition #olympiad #matholympiad #mathematics #sybermath #aplusbi #shortsofsyber #iit #iitjee #iitjeepreparation #iitjeemaths #exponentialequations #exponents #exponential #exponent #systemsofequations #systems
#functionalequations #functions #function #maths #counting #sequencesandseries
#algebra #numbertheory #geometry #calculus #counting #mathcontests #mathcompetitions
via @RUclips @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Number Theory Problems: • Number Theory Problems
Challenging Math Problems: • Challenging Math Problems
Trigonometry Problems: • Trigonometry Problems
Diophantine Equations and Systems: • Diophantine Equations ...
Calculus: • Calculus
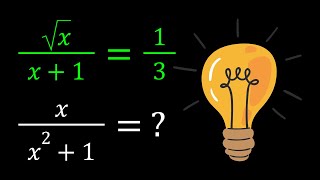








Saw the substitution you showed off in your second method pretty quick, I'm happy I'm understanding the trick behind these more and more often
Great to hear!
Yep, method 2. After I substituted x = u*sqrt(12) on the RHS, I saw how the LHS simplified and nicely fell in line. How convenient. LOL
The trick to add the coefficients can be used to quickly see if x=1 is a root. You can also use Descartes’ rule of signs to get a good idea how many positive snd negative roots there are. All of which can reduce the number of trials for the rational root theorem. With synthetic division/substitution you can also see when the remainders change sign for successive candidate roots, telling you a root is between those candidates.
Good thinking
let a=x/3, b=4/x, i.e. ab=4/3
the equation becomes: 3a^2+3b^2 = 10 (a-b)
a^2+b^2 = 10/3 * (a-b)
a^2 - 2ab +b^2 +2ab = 10/3 * (a-b), want to make (a-b)
(a-b)^2 - 10/3 * (a-b) + 8/3 = 0
solving (a-b) = 2 or 4/3
put back x into a & b, solve the 2 quadratic equations, then you have 4 answers
Multiply both sides on 3.
x^2 + 144/x^2 = 10 ( x - 12/x)
x - 12/x = t
I seems tome that is easier way.
Do you see what I see? 😊
Thank you
Re "But it's a contrived problem" at 9:00: Thank God for contrived problems!
Otherwise just ask first graders to solve the Bohr hydrogen atom - as that too is just a contrived problem.
Thanks
My solutions are x=-2,6,3+-√21.
x^4 - 10x^3 + 120x + 144 = 0
x^2 - 10x + 120/x + 144/x^2 = 0
(x - 12/x)^2 - 10(x - 12/x) + 24 = 0
Let t = (x - 12/x)
t^2 - 10t + 24 = 0
t = 4 or t = 6
etc.
x^2/9+16/x^2=10/3(x/3-4/x) set t=(x/3-4/x) t^2+8/3=10/3*t 3t^2-10t+8=0 (3t-2)(t-4)=0
La ambele metode ,solutiile sunt :-1 ;-2 ; 6 ; 7 dar verifica doar -2 ; 6. A doua metoda mi-a plăcut mult!
First i tried multyplying both sides with 1/4 to get (x^2/12)+(12/x^2)=(10/4)*[(x/12)-(1/x)]=? =10*[(x/48)-(1/4x)]........? Then tried multyplying with 3 and got x^2+(144/x^2)=10*(x-12/x) EQ(1) and setting x-12/x=t also raising both sides in 2nd, we get x^2+(144/x^2)-24=t^2 so x^2+(144/x^2)=t^2+24 . The equation (1) becomes t^2+24=10t so t^2-10t+24=0 so t=(10+ -sqrt4)/2 so t=5+ -1 so t=6 or t=4 (2). CASE 1 t=6 so x-12/x=6 so x*2-6x-12=0 so x= (6+ -sqrt84)/2= (6+ - 2*sqrt21)/2= 3+ -sqrt21 (3) . CASE (2) t=4 so x-12/x =4 so x^2-4x-12=0 so x=(4+ - sqrt64)/2=(4+ -8)/2=12/2 or -4/2 so x=6 or x=-2 (4). Combining (3) and (4) we have 4 solutions x= -2, x=3-sqrt21, x=6, x=3+sqrt21 in ascending order.
Comedy
You are doing too much work with your first method which you didn't finish. At 1:45 you have arrived at the quartic equation
x⁴ − 10x³ + 120x + 144 = 0
Unlike what you seem to suggest, there is no need to depress this quartic in onder to solve it using Ferrari's method. Bringing all terms of a lower than the third degree over to the right hand side we have
x⁴ − 10x³ = −120x − 144
In order to complete the square at the left hand side we note that x⁴ = (x²)² is the square of x² and that 10x³ = 2·x²·5x is twice the product of x² and 5x, so we need to add (5x)² = 25x² to both sides to make the left hand side into a perfect square x⁴ − 10x³ + 25x² = (x² − 5x)². This gives
(x² − 5x)² = 25x² − 120x − 144
We now need to add something to both sides in order to make the right hand side into a perfect square as well, but we need to do this in such a way that the left hand side will remain a perfect square. If we take any number k and add 2k(x² − 5x) + k² = 2kx² − 10kx + k² to both sides, then the left hand side (x² − 5x)² + 2k(x² − 5x) + k² = (x² − 5x + k)² will remain a perfect square regardless of the value of k. Adding 2k(x² − 5x) + k² = 2kx² − 10kx + k² to both sides we therefore have
(x² − 5x)² + 2k(x² − 5x) + k² = 25x² − 120x − 144 + 2kx² − 10kx + k²
which gives
(x² − 5x + k)² = (2k + 25)x² − (10k + 120)x + (k² − 144)
The left hand side is a perfect square for any value of k, so we are now free to choose k in such a way that the quadratic in x at the the right hand side will also be a perfect square. A quadratic ax² + bx + c is a perfect square, i.e. the square of a linear polynomial in x, if and only if its discriminant b² − 4ac is zero, so the condition which k must satisfy in order for the right hand side of our equation to become a perfect square is
(10k + 120)² − 4(2k + 25)(k² − 144) = 0
This is a cubic equation in k known as the _resolvent_ of our quartic equation but, more often than not, we do _not_ need to solve this equation formally. This is so because quartic equations with integer coefficients which arise in Olympiad problems mostly have nice factorizations into two quadratics with integer coefficients.
If x⁴ − 10x³ + 120x + 144 can indeed be factored into two quadratics with integer coefficients, then there must exist a value of k which makes the right hand side (2k + 25)x² − (10k + 120)x + (k² − 144) of our quartic equation the square of a linear polynomial such that at least the coefficient 2k + 25 of the quadratic term is the square of an integer. Therefore, in order to find a potential factorization into two quadratics with integer coefficients we only need to check values of k which make 2k + 25 equal to 1, 4, 9, 16 ... which substantially limits the values of k we need to try.
In fact, for the very first possibility 2k + 25 = 1 we must have k = −12 and with this value of k both the coefficient −(10k + 120) of x and the constant term k² − 144 at the right hand side reduce to zero. So, with k = −12 our equation becomes
(x² − 5x − 12)² = x²
where both sides are now a perfect square. This equation is easily solved using the equal squares property A² = B² ⟺ A = B ⋁ A = −B which gives
x² − 5x − 12 = x ⋁ x² − 5x − 12 = −x
x² − 6x − 12 = 0 ⋁ x² − 4x − 12 = 0
(x − 3)² = 21 ⋁ (x − 2)² = 16
Noting that 21 = (√21)² and 16 = 4² and again using the equal squares property this gives
x − 3 = √21 ⋁ x − 3 = −√21 ⋁ x − 2 = 4 ⋁ x − 2 = −4
x = 3 + √21 ⋁ x = 3 − √21 ⋁ x = 6 ⋁ x = −2
Alternatively, we can bring the square from the right hand side over to the left hand side to create a difference of two squares at the left hand side whereas the right hand sides becomes zero so we get
(x² − 5x − 12)² − x² = 0
Then, we can use the difference of two squares identity a² − b² = (a − b)(a + b) to factor the left hand side into two quadratics which gives
(x² − 6x − 12)(x² − 4x − 12) = 0
and the zero product property AB = 0 ⟺ A = 0 ⋁ B = 0 then gives the same two quadratic equations. Of course, the two approaches are equivalent because the equal squares property is a simple consequence of the difference of two squares identity and the zero product property:
A² = B² ⟺ A² − B² = 0 ⟺ (A − B)(A + B) = 0 ⟺ A − B = 0 ⋁ A + B = 0 ⟺ A = B ⋁ A = −B
😎✌️😃👏👍😃👏👍👏😎
x^4-10x^3+120x+144 --> , (x+2)(x^3-12x^2+24x+72)=0 , x=-2 , x^3-12x^2+24x+72=0 --> , (x-6)(x^2-6x-12)=0 , x=6 ,
x^2-6x-12=0 , x=(6 |+/-| sqrt84)/2 , x= 3+V21 , 3-V21 , solu. , x= -2 , 6 , 3+V21 , 3-V21 ,
(x/√3-4√3/x)^2+8=(10/√3)(x/√3-4√3/x)...t^2+8=(10/√3)t..t=5/√3+√(1/3)=6/√3=2√3….(t=4/√3)..x/√3-4√3/x=2√3...x-12/x=6..x^2-6x-12=0..x=3+√21..x=3-√21...x/√3-4√3/x=4/√3...x-12/x=4...x^2-4x-12=0...x=2+√16=6...x=2-√16=-2