Solving Jensen's Functional Equation
HTML-код
- Опубликовано: 30 июн 2024
- Join this channel to get access to perks:→ bit.ly/3cBgfR1
My merch → teespring.com/stores/sybermat...
Follow me → / sybermath
Subscribe → ruclips.net/user/SyberMath?sub...
Suggest → forms.gle/A5bGhTyZqYw937W58
If you need to post a picture of your solution or idea:
intent/tweet?text...
#ChallengingMathProblems #FunctionalEquations
PLAYLISTS 🎵 :
Number Theory Problems: • Number Theory Problems
Challenging Math Problems: • Challenging Math Problems
Trigonometry Problems: • Trigonometry Problems
Diophantine Equations and Systems: • Diophantine Equations ...
Calculus: • Calculus


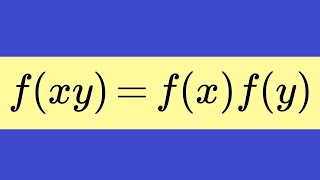





There are infinitely many other functions that satisfy Cauchy's functional equation. You must supply additional conditions on f
Consider R as a vector space over Q. It has a basis. Then f is uniquely defined by its values on the basis up to a constant and these values can be arbitrary. For f to be linear you need either continuity or positivity assumption (f(x)>0 if x>0).
It's simple to see that any constant would work, and that any constant times x. You see that you can make any linear combination of those, and thus, it is trivial to see that every function ax+b would work. Now proving no other functions do is a whole different part
Well, obviously those are not quite all the solutions, there are pathological solutions similar to the ones to Cauchy's Function Equation
At 7:40, you reasoned because g(w+z)=g(w)+g(z), then g must be linear. If that is so, couldn’t you have concluded the same thing about f from the very statement of the problem: f((x+y)/2)=(f(x)+f(y))/2?
How did he conclude that g is linear? A Didnt get it
@@cau1834 g is linear only if restricted to rational numbers, thus supposing additionaly that f is continuous for example, you can conclude the linearity of g.
@@danielvieira8374 Thankyou! I had overlooked that this conclusion implicitly assumed continuity. Much appreciated!
If f:X-->Y is continous defined between 2 Banach spaces then f is linear. Since the set of all fraction of the form m/2ⁿ is dense in [0, 1] .
I like functional equations and am planning to cover many of them. I like how we can be a bit more creative with them. Nice work! Enjoyed this video so much Sybermath!👍👍👍
Thank you Dr PK Math! Functional equations are fun!!!
Amazing video and solution. I love it!!!
Glad you like it! 🤩💖😊
Much easier solution: Visual inspection of the original equation shows that f(x) must be linear, so f(x)=mx + b
Proof of linearity from it's definition would be fine addition.
@@JeremyGwag You can't prove it, there are other solutions, admittedly they are pathological, meaning there are not "nice" at all, not useful, and if you impose some very weak restrictions then the only solutions are linear polynomials
from the equation we see that the function must be scalar multiplicative and linear and thus must be of the form ax+b
from Jensen's inequality it can be shown that f''(x)=0 for all x. Therefore f(x)=mx+k
Differentiability is not given, you can't assume it
@@anshumanagrawal346 But we do not need it. Just look if f is conves or concave without f''
@@bartekabuz855 Doesn't make sense to talk about convexity of functions that aren't differentiable, much less ones that aren't continuous
Beautiful!
Thank you! 😊
A function satisfying g(x+y) = g(x) + g(y) for all real x, y does NOT necessarily linear over the field R of the real numbers (it does over the field Q of the rational numbers); Thus you CANNOT deduce g(x) = ax for some a from that equation.
*Looks*. It’s linear. *Done*
f[(x+y)/2]=(1/2)*[f(x)+f(y)].
A short solution for those who understand.
Differentiate both parts of the equality by x (consider y constant):
[f(u)/du]*d[(x+y)/2]/dx=(1/2)*df(x)/dx +0, u=(x+y)/2. From which we obtain:
df(u)/du=df(x)/dx (1)
Similarly, differentiate the original equality by y, and we obtain,
df(u)/du=df(y)/dy (2)
From (1) and (2) df(x)/dx = df(y)/dy (3)
The left side of (3) depends only on the independent variable x,
and the right only on the independent variable y. This is only possible if
df(x)/dx = const. To make the author pleasant, we denote her m.
Then, f(x)= mx+k
Let's make the author pleasant once again, and denote the second indefinite constant that occurs during integration as "k".
If f is a solution it is easy to see that x---> f(x) + constante is also solution. we could assume f(0) = 0
y=0 ---> f(x/2) = f(x)/2 ----> (f(x) + f(y)) / 2 = f((x+y)/2) = (1/2)* f(x+y) = ----> f(x) + f(y) = f(x+y) Cauchy equation
We know that the solutions of Cauchy equation are linear functions ----> f (x) is linear function ---> f(x) = ax + b
Remark : Let g(x) = f(x) + k---> g((x+y)/2) = f((x+y)/2) + k = f(x)/2 + k/2 + f(y)/2 + k/2 = 1/2 *(f(x) +k) + 1/2 *(f(y) +k)
g((x+y)/2) = 1/2 *g(x)+ 1/2 *g(y)
In general, it is incorrect that the solutions of Cauchy equation are linear functions
@@Buluar Cauchy Functional Equation
Cauchy's functional equation is the equation
f(x+y)=f(x)+f(y).
It was proved by Cauchy in 1821 that the only continuous solutions of this functional equation from R into R are those of the form f(x)=kx for some real number k. In 1875, Darboux showed that the continuity hypothesis could be replaced by continuity at a single point and, five years later, proved that it would be enough to assume that f(x) is nonnegative (or nonpositive) for sufficiently small positive x.
In 1905, G. Hamel proved that there are non-continuous solutions of the Cauchy functional equation using Hamel bases. Every non-continuous solution is necessarily non-measurable with respect to the Lebesgue measure.
The fifth of Hilbert's problems is a generalization of this equation.
Another way to find the same solution is the following:
First try to restrict f to integer values and find its value expressed with a formula, then prove that the values of f for non-integer values x must be equal to the value of the same formula at x.
Substitute x=k+1 and y=k-1, for some integer k: f(k) = (f(k+1)+f(k-1))/2. This is a recursion in k, and can be solved to give: f(k) = k*(f1-f0) + f0. So f(x) = x*(f1-f1)+f0, where f1=f(1) and f0=f(0) are any freely chosen real values.
To show that this extension must hold for all real values x, consider the following:
f(x) = f([x]+{x}) = f((2[x]+2{x})/2) = f(2[x])/2 + f(2{x})/2 = [x](f1-f0) + f0/2 + f(2{x})/2 = ([x]+{x})(f1-f0) + f0 = x(f1-f0) + f0, where [x] and {x} are the integral and fractional parts of x, respectively.
To prove that this holds, it still has to be shown that f(2{x})/2 = {x}(f1-f0) + f0/2.
Nice and good, I am a great fan of Maths.
Very nice 👌
Thanks 😊
An operation that preserves the mean. That is mean(f(x + y)) = mean(f(x) + f(y)) = mean(f(x), f(y)). According to my intuition, this has to be a linear function f(x) = Ax. Are there other solutions? This is Cauchy's functional eq right?
I guessed f should be a linear function taking into account the graphical representation of functions. But there was no way I could prove this analitycally. Just a question, do linear functions include constant functions? I am asking this because any constant function is also a solution.
f(x)=mx+b is constant for m=0
"I hope you enjoy it, please let me know"
You'll know, I enjoy it
💖🤩
This equation is the not-convex and not-concave case of Jensen's Inequality. In other words, some line in the plane.
Gotta know the difference between divide and multiply
Can you please say the book name of the source question? Reply please
I don't remember the name of the book but this equation is pretty common and known as Jensen's functional equation. Check this out (page 5):
web.evanchen.cc/handouts/FuncEq-Intro/FuncEq-Intro.pdf
My guess was also linear but i made some mistakes
First prove f(0) = 0, then prove f(2x)=2f(x), then f(x+y) = f(x) + f(y).
Aren't you also supposed to proof that g(kx) = k*g(x) for all k and x in order to assume g to be a linear function?
Edit: oh wait I'm dumbo nevermind that's not how it works for linear functions
😁
just do a differentiation and you got linear relationship of dx and df
Can you elaborate?
@@SyberMath let Y=0. F(x/2) = f(x)/2 + f(0)/2. f(0) is a constant per any f(). so let x/2=a. f(a) = f(2a) /2 + c. diff both side of a, f'(a) = 2 f ' (2a) for all a's. so use the proof of contradiction, for any d(a) trend f'(a) is not increasing, not decreasing so f'(a) has to be flat. so f'(a) is a constant. so f(x) is kx+f(0). done.
Can't we arrive at the conclusion that f(x) must be linear if f(x)+f(-x)=2f(0)? because if we assume more degrees of polynomial than 2 then the term x square, X power 4 will remain for increasing powers?
Do we know if f is a polynomial?
@@SyberMath Oh I hadn't thought about that nice. But if we assume that f is polynomial then only my thinking will work so technically I went along the route of misconception.
@@SyberMath This was a very good problem. I thoroughly enjoyed the substitutions throughout the video. I suggest you to post more problems of equal or more rigour.
Very nice problem
c+x/2
Hi Zhard Chang ☺
@@jimmykitty hi, kitty
Let v be the average of x and y. The slope between (x, f(x)) and (v, f(v)) is
(f(v)-f(x))/(v-x)
= (f(x) + f(y))/2 - f(x))/(v-x)
= (f(x) + f(y) - 2f(x))/(x + y - 2x)
= (f(y) - f(x))/(y - x) = the slope between (x, f(x)) and (y, f(y))
It can similarly be shown that the slope between (v, f(v)) and (y, f(y)) also equals the slope between (x, f(x)) and (y, f(y)).
Because the slope between any pair of points is the same, (x, f(x)), (v, f(v)), and (y, f(y)) are always collinear; they must lie on a linear function.
Now, how to prove that equal slopes must imply collinearity...
This is actually really smart, nice solution
Nice!
@@SyberMath You say it's a common technique, but i don't see why anyone would think of this to begin with if they didn't already know it was a technique..not even a supposed math whiz..wouldn't you agree?
@@SyberMath Seriosuly i dont see ANYONE EVER EVER doing thjs why introduce knand g it's not necessary..if you want bit don't youbagree respectfully it's needlessly convoluted..
I am not a maths student:-)
Don't most ppl.think this is needlessly convoluted??
You missed infinitely many solutions. For example, f(x)=2x if x is rational, 0 is it is not
f(4+π)=f(4-π)=0 but f(4)=8
30 seconds problem stretched into 10 mins
Really?
@@SyberMath if f((x+y) /2)=(f(x)+f(y))/2 it is a straight line. Full stop.
Is there an example to where functional equations will be useful in real life?
Nope
@@jett23900 weeb
Don’t worry, you won’t need them. Only smart people have to deal with them.
@@fullfungo "I don't know" would have been enough.
Being bases of iterative methods for numerically approximating solutions of equations, for instance.
Solution too long.
I woke up in the middle of the night,, saw the problem and solved it mentally in 10 seconds before watching the video.
The image of the average of values is equal to the average of the images of the two values
=> f is linear, i.e., f(x)=ax+b
In fact, differentiating on both sides of the equation over x with y as parameter independent of x:
=> 1/2*f’[(x+y)/2] = 1/2*f’(x)
=> f’(x)=f’[(x+y)/2] for any y.
=> f’ is constant
=> f(x)= ax+b
b can be any number since by shifting line along the y axis, the images of the teo values and their center all shift by the same value.
This can also be verified.
For y=0, original equation gives
f(x/2)=f[(x)+f(0)]/2
=> ax/2+b=[ax+2b]/2
=> ax/2+b = ax/2+b always true
=> solution: f(x)=ax+b
I didn't nothing! And you?
Hai, Are you from Ukraina ?
Hi. No I'm not