Integration by parts two times (KristaKingMath)
HTML-код
- Опубликовано: 9 фев 2025
- ► My Integrals course: www.kristaking...
Learn how to use the integration by parts formula to find the integral of a function involving the exponential (e^x) and a trigonometric function. To complete this problem, you'll need to identify which part of the function will be set equal to u, and which part of the function will be set equal to dv. Then you'll take the derivative of u to find du and take the integral of dv to find v. Then you'll plug u, du, v and dv into your integration by parts formula. With this particular problem, you'll have to perform a second round of integration by parts. After using integration by parts twice, you'll have to move the remaining integral from the right hand side to the left hand side, and then divide both sides by the coefficient from the left hand side in order to solve for the original integral.
● ● ● GET EXTRA HELP ● ● ●
If you could use some extra help with your math class, then check out Krista’s website // www.kristakingm...
● ● ● CONNECT WITH KRISTA ● ● ●
Hi, I’m Krista! I make math courses to keep you from banging your head against the wall. ;)
Math class was always so frustrating for me. I’d go to a class, spend hours on homework, and three days later have an “Ah-ha!” moment about how the problems worked that could have slashed my homework time in half. I’d think, “WHY didn’t my teacher just tell me this in the first place?!”
So I started tutoring to keep other people out of the same aggravating, time-sucking cycle. Since then, I’ve recorded tons of videos and written out cheat-sheet style notes and formula sheets to help every math student-from basic middle school classes to advanced college calculus-figure out what’s going on, understand the important concepts, and pass their classes, once and for all. Interested in getting help? Learn more here: www.kristakingm...
FACEBOOK // / kristakingmath
TWITTER // / kristakingmath
INSTAGRAM // / kristakingmath
PINTEREST // / kristakingmath
GOOGLE+ // plus.google.co...
QUORA // www.quora.com/...
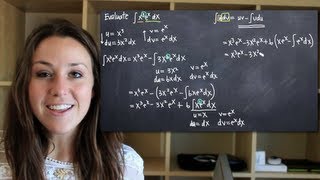








I used to watch your videos when I was taking AP calc in my high school days and now I came back for more advanced calc. I cannot thank you enough. Your explanations are detailed, concise, and considerate. Thank you so much for the videos.
I have been teaching for more than 38 years. You are amazingly good and efficient. Thank you. The Planetary Society Argentina.
I'm so glad you like my teaching style! :)
engineering student here... clicked subcribed so fast xD
+Danish Fiesta LOL, nice!
Krista King hey I want speck with u
This BLEW MY MIND! I was like "no way is this getting simpler!" Then BAM
You have no clue how you saved my Cal 3 grade .Thank you for your service.God Bless you in every way possible
I'm so glad I could help! 😊
thank you GOD for having someone with enthusiasm and the ability to teach/instruct WITHOUT a monotone voice. The fact that you reiterate why you do certain steps is why I can follow along :]
I'm so glad the videos are helping!! :D
Even after completing 9 years this video remains very helpful to all those who struggle at calculus.
Best explanation I've ever seen ! Clear and precise, btw your voice is wonderful for teaching Math : D
Best step by step of Integration By Parts on the internet. Thank you Krista!
You're welcome, Jordan! I'm so glad you liked it!! :D
night before my exam and had no idea what this was called but through a lot of google searches I found this and thank goodness, you are a life saver! I owe you so much!
i'm so glad you found this, and that it helped clear things up! :D good luck on your exam!! :D
I'm glad I can at least teach on RUclips and help people all over the world that way. :)
In this problem, the choice of u and dv don't matter because neither e^(7x) or cos(2x) become appreciably simpler when integrated or differentiated (adds a constant of 7 or 1/7 in the e^(7x) and adds a -2 or a 1/2 factor and changes cos to sin in cos(2x) case)
for me it's mostly about gaining an intuition through a lot of practice, but the LIATE rule can also give you a decent idea. it tells you to pick a Logarithmic function, an Inverse trig function, and Algebraic function, a Trig function, or an Exponential function, in that order, if you have one of them in your integral. hope that helps! :)
Thank you for being old-fashioned (i.e. mathematically rigorous) enough to take that last step to factor the answer. Too many other math RUclipsrs would simply leave it unfactored. This in itself could mark the difference between a five versus a four on the AP exams.
:D
Cal 2 is so difficult. People are dropping like flies in my class. Thank GOD you are posting these videos.
You're putting in the extra work, and it's paying off! I'm so glad I can help along the way. :)
Thank you so much,I have differential equation exam in 12 minutes and you explained this beautifully. It's been 8 years, I hope you are in good health and doing great in life.👏👏
Best explanation ever! Honestly RUclips and a bunch of great teachers like you have been my source of study for the past two years (way better than school textbooks and teachers sometimes with all due respect), I couldn't be more grateful! ^_^
I'm so glad I've been able to help along the way! Thanks for letting me know. :)
^_^ Starting to enjoy calculus and algebra now :p gonna keep commenting because they're all useful, hope you don't mind that hahaha :)
Ted Tang I don't mind at all!
Yay! :p
One of the best IBP explanations I've come across.
Thank you so much, Skool! :)
This really helped my understanding of how to integrate by parts. Something my teachers haven't been able to do so far, so thank you a lot! :) You just made my future studies much easier
I'm glad I can help! :D
I'v learn't in your videos ever than any other clips have come by. You're really doing a good job explaining every little details of your steps. Most especially whenever you do a quick flash back- that kind of safe a lot stress, refreshing ones memory of what is forgotten. And of course a sure savior of terrible prof. I followed your channel already. Keep doing what you are doing.
I'm so glad you're liking the videos! Thanks for the comment. :D
Always my pleasure integralCALC
This is such a clever way to solve integrals. I just learned integration by parts today. Interesting.
wow! who would have guessed how the (-) integral term of the second integration by parts would look so much like the original integrand!??!!! And how it all comes out like it does- yippee! Prof. Krista makes it look easy! - or at least possible... three cheers for Prof. Krista! Thanks!
finally my confusion on Integration by parts has been removed by watching your amazing video. you are too cool !!!..keep it up...
+Sourav Ahmed I'm so glad it helped!
This is a very informative video I must say. Thank you so much for all your valuable time
You're welcome, I'm so glad you liked it!
i used to tkae this sub,,last semester and i got F,,,and i am taking again this semester,,,by watching your video,,now i can solve problems,,
i'm so glad the videos are helping, and i hope you do much better this semester!
integralCALC thanks....
U r so good teacher and i wana ur type of teacher.....u explain everything so politely like mother deals with her baby.....
And i love ur these type of expressions...
Love u....😘
Never knew about the trick you can use if you end up with the same integral as you started with, super cool! Thank you
you bet! :D
You're my new tutor, your videos are awesome!
:D
I was getting stuck at the end of the 2nd Integration by parts, but this video helped me notice how to substitute it back in and add to the other side. Thank you so much!
I'm glad it could help!
You really smart , I understand and think I could do a similar problem.
I like how you analyze the problem before you start !
Makes so much more sense after it is evaluated at this pace. Thanks so much for explaining every step, something my high school calc teacher should take notes on lol
I'm so glad it helped!
Fantastic format and video. You're now my Favorite RUclips math instructor:)
In terms of format, you must have taken a page from Derek Owens. Derek now has a competitor. Great, fantastic work, both of you! ("Pat" too).
+hettygreene Thank you! I had never heard of Derek Owens before you mentioned him, but you're right, our video formats do look similar.
If I could build a flower out of integrals and derivatives you'd be the first one I'd give to you. But since I can't I give you a high five for the great examples ^_^
This video was extremely helpful, I learned it so quick and you explained just how I felt with going right back to where I started. Bravo!
Thanks Nicko, I'm so glad it helped! :)
Miss ,you have helped me alot in my practice of integration ,i can't thank you enough. Im having paper of Maths on 5th May .Me and my friend ,we have practiced everything from limits,derivatives ,Conics, analytical geometry and the mighty " integration " but yet we feel nervous and tense from keeping ourselves away from mistakes . We hope that paper will be easy ,i just pray everything goes smoothly . Please Miss pray for me and my friend to get success and victory in maths paper......☺
+Hamza Khan I hope that everything goes great for you guys! Just try to take your time and stay focused, and you'll do great!
You seriously have the best explanations. Much appreciated.
Your a total whatababe! Thanks for the video, concise and easy to understand. Keep up the good work !
I understand more now than I do in class. very helpful! thank you for posting
+stephsoprty You're welcome, I'm so glad it helped!
i was doing that dumb thing of taking by parts again and again all the way upto 4th time....frustrated...came here and BOOOM!!!!....you just solved my problem in an instant......Thankyou so much.....
You're welcome, Muhammad! I'm so glad this cleared up why it wasn't working! :D
thank you so much, way easier to understand than how we were taught in our uni lecture. Much love
Glad I could help! :D
You're so welcome! Glad I can help! :)
Extremely helpful tutorial. Not surprised that you have over 48,000 subscribers. Here's another!
awww thank you so much!
You are amazing! I have been watching your videos for a few days now, and you seem to make it soo much easier! I wish I had started viewing them sooner though. I've struggled a lot this semester with my calculus class (I live in Sweden and evidentily our professors can't explain anything..) So I have to retake my calculus exam next week. Thanks to you I think I might have a chance of passing! So thank you a million times and keep up the good work!
I'm sorry to hear that this semester's been tough, but I'm so glad the videos have been helpful! I hope they'll be just the thing to get you past your exam next week. :) Good luck!! :D
Yes it's been hard. I have one question though and I'd be so happy if you could answer it. I watched another clip on youtube about a double integral. The function was 2y/(x^2+1) dydx. The guy who did this integral said that if you integrate over y first, and then have to do a u-substitution to integrate over x, you have to change the limits of integration for x. For example the limits were first x=0 and x=1, he was integrating x/(x^2+1) for x, substituting u=x^2+1,du=2x dx. So he said that you'd have to put the limits of integration for x (x=0 and x=1) into u=x^2+1, which gives the new limits of integration x=1 and x=2. I have never seen this before! Is this something you'd do? I hope you understand what I mean! :)
Helena engström I understand exactly what you mean, and yes, that's something you can do, but you don't HAVE to. Whenever you're doing a u-substitution, you always have two options. 1. You can plug the original limits of integration into the equation for u to get new limits of integration in terms of u, instead of in terms of x. Then, once you integrate, you can plug the limits of integration directly into the integrated function, without back-substituting, because your function will be in terms of u and your limits of integration will be in terms of u. 2. You can leave the original limits of integration as they are, in terms of x, and then after you integrate, and your function is in terms of u, you have to back-substitute for u to get your function back in terms of x. That way, you'll have a function in terms of x, and the original limits of integration in terms of x, and you'll then be able to evaluate over the original limits of integration. Hope that helps! :D
Yes that helped a lot! Thank you so much again! :D
Hi there! I think there is a simple saw to solve this problem without using integration by parts. Using complex number would be a big help. We use Euler's formula for cos(2x) = 1/2* (e^(2ix)+e^(-2ix)). We now have a series of exponential functions to integrate. Thanks
I've watched videos from Khan Academy. Yours are much better.
Thankyou so much , you are changing the world one student at a time
I'm so happy I can help! :)
So glad you like the videos! :)
I love all your videos. Thank you for your effort, care, and patience.
Aw thanks! I really appreciate it.
Krista you are amazing. Thank you for posting these videos!
Aw thanks Amelia!
Glad you like the videos! :)
Krista 👑 you Saved my life... that was quite easily done ✅ thank you so much....
Glad it could help!
Thank you so much for your video , it is really helpful and so clear for us . I really appreciate your efforts to explain this video very well . Well done :)
Thanks for letting me know! :)
very superior transfer of the subject from your mind to my mind
I had the exact equation in my homework due today. Missed it by a mile...I’m subscribing.
Thank you so much for the sub, Drew! :D
Your video is very helpful, Thank you very much for making it very simpler !!!
You're welcome, I'm so glad it helped! :D
I enjoy your videos, they have helped me through math. Thanks.
I'm taking calc 3 after taking a semester out for linear algebra, so this came in really handy this evening - thanks!
Yay! I'm so glad it could help. :)
integralCALC Are you currently seeing anyone ;)
These calculus related problems have become like the alphabet now. Thanks for your demonstration showing techniques of making the 'u" the trigs part, and dv the exponential part in the formula integral udv = uv - integral vdu. for an easier outcome
you're welcome! i'm glad it helped. :)
Thank you so much for your inspiring mathematical enhancement. You dismiss the theory that girls are weak at maths while simultaneously projecting brain and beauty. Please do enhance my song She figured it.....youtube...you deserved more appraisal. also please note suggested change in line 'Legs design for romancing' instead of 'begging'...And the integration by parts of the components of the body into one whole harmonious beauty enhanced by integral CALC.. Best Regards.
lovely its so clear and makes maths simple
Nice, i wish every calculus class was like this!
I'm so glad I could help! :)
You are awesome!!! This was extremely helpful and now I understand it much better. Thanks a bunch :-D
tjustice808 You're welcome! Glad I could help!
thank you very much for your effort ! your tip's , way , and your calm way in solving equation's is just a joy for me to study calculus , you helped me ALLOT !!!
thanks!! i'm so glad you liked it!! :D
This had helped me strengthen my weaker portions...Thank you!
+Waleed Khan You're welcome, I'm happy it helped!
Coming back to this after a while, it seems to me that pulling out the exponential term and factorising at the end might be the best thing in an engineering context, as it more clearly delineates oscillatory vs. damping/divergent terms in any system this equation might describe. (Obviously with e^7x terms in the numerator it's going to blow up fairly quickly, but in systems whose coefficients or whose coefficients on exponents are symbolic of physical inputs under the engineer's control, it can point the way to stabilising the system.)
Ahhhh! now i see :D
8 years on and still helping us !!
I'm so glad the video helped, stuart! :)
Thank you very much. I am really having a terrible time understanding this topic. But you have made it easier for me. Thanks! :))))
I'm so glad it's getting easier for you... that makes me so happy!! :D
I'll be making some more in near future! :)
You make math even more fun than it lready is :-) Thanks !
Great job explaining this, definitely helped me prepare for my Calc BC test!
you are now officially important in my life.
Right back at ya Craig! :D
This is really good. very well paced and well delivered. good luck :)
Thanks! :)
geez! thank you so much. I guess now I'll be able to correct my calculus professor whenever we'll learn this.
excellent! you just saved my exam! much love
I hope you rocked the exam! :)
had an 85%
Great job!! That's awesome! :D
You are talented at communicating!
Thank you!
Thanks!
I've never found integration by part so attractive until now.
Splendid !!! u r so nice and helpful god bless you whatever your name is
omg everybody who's watching this video is taking calculus lessons at uni but here i am 17 years old and have to deal with this insane math in high school and expected to solve this and anothr 49 questions like that in 80 minutes to go to the college. great education system us asians have. really.
Very clear explanation, thank you!!!!
Thanks! Glad you liked it! :)
That was a beast of a question!
Very clear and understandable evaluation. Thank you :)
+Guus Korver Thanks!
I did very well on my exam :D
A+ for maths c and B+ for maths b
I love you!! Come take over my Calculus 2 class please lolol!!! You do your job wonderfully!!
+Dylan Joseph lol, at least I can help through the videos!
10:00
Holy mother....f....king....you just blew my mind when you added that nasty integral to the other side!!!!!!!!!!!!!!!! WoW
Thank you so much for this video. It helped me out so much.
Awesome! Thanks for letting me know.
thank you so much! i have a final in two days and this was very helpful... and you are very beautiful just makes it that much better :)
Good luck on the final!
like u maam u make me refresh my calculus
Nice mam....am from india but u r voice clearly understood....
Thanks, Ganesh! I'm so glad it made sense! :D
thank you for this video. because yes - @ 9:55 - that is where i totally get discouraged and feel like i've made no progress - or, by that point i have butchered my algebra so bad that the problem is no longer recognizable.
+Chance Wilson I think we've all felt that way doing these kinds of problems... so you're not alone! The more you practice them, the less overwhelming they feel, so just keep going... you're doing great!!
You explain these concepts so well... I think I am good in mathematics ... I can do this integral easily. ...however if someone asks me to explain it , I will look like a fool . ... seriously I am a horrible tutor... good 4u!...
lol, thank you!
I saw your explanation on newtons method but I find that one a little more challenging. ..
ura239 it'll get easier, you just have to get the hang of it!
I really want to get it down ,simply because it's newton's method ....lol....if that makes any sense. .... I'm curious to know if you are a professor or a student.
ura239 Neither! I'm a tutor.
Thank you soo much you just gave me hope for my calc test
DJ Sandhu I'm so glad! Good luck on your test, I hope it goes great!!
perfect explanation... thank you so much
Great video. Helped me a lot. Thanks
Good heavans to mercy!!! Why did I take calc 2? I'm spazzing out right now but you helped tremendously!!!
Brilliant tutorial!!! Thanks!
Thanks a lot for this video, you made it crystal clear.
+Ryan Durbin Awesome! Thanks for the feedback.
Excellent explanation , thanks !
Thanks! :)