
- Видео 34
- Просмотров 106 652
n choose k
США
Добавлен 11 июл 2019
Thank you for visiting my channel! My name is Kuang. I am currently a Ph.D. student in Mathematics at UCSD, and I am also a dad of a beautiful 2 year old.
I have a huge passion in teaching so I use RUclips as a platform to share my knowledge to you. I make videos going over various math topics, including Calculus, Linear Algebra, Combinatorics, and more. My main goal is to share problem solving tricks I have learned throughout my teaching career to public. Tricks that you cannot find anywhere else!
Please subscribe so you won't miss my latest video!
I have a huge passion in teaching so I use RUclips as a platform to share my knowledge to you. I make videos going over various math topics, including Calculus, Linear Algebra, Combinatorics, and more. My main goal is to share problem solving tricks I have learned throughout my teaching career to public. Tricks that you cannot find anywhere else!
Please subscribe so you won't miss my latest video!
Stephen Curry made 105 straight 3-pointers || How good he actually is.
December 24 2020, a two-time NBA MVP Stephen Curry made 105 3 point shots in a row. Luckily, his teammate had that recorded or no one would believe that. Of course, we all know that Stephen is really good at that, but to be able to make 105 in a row... we analyze how good he actually is in this video.
Video mentioned:
Steph making 105 in a row: ruclips.net/video/1mi-lCTCvrE/видео.html
Steph 94/100 3 pointers in 2018: ruclips.net/video/nXCol8YeqxQ/видео.html
Fred Newman 209 in a row: ruclips.net/video/NzYh4CGAbCU/видео.html
Facebook: N-choose-k-RUclips-105387694184879/
Twitter: KuangSittipong
Website: www.math.ucsd.edu/~sithamro/
Video mentioned:
Steph making 105 in a row: ruclips.net/video/1mi-lCTCvrE/видео.html
Steph 94/100 3 pointers in 2018: ruclips.net/video/nXCol8YeqxQ/видео.html
Fred Newman 209 in a row: ruclips.net/video/NzYh4CGAbCU/видео.html
Facebook: N-choose-k-RUclips-105387694184879/
Twitter: KuangSittipong
Website: www.math.ucsd.edu/~sithamro/
Просмотров: 723
Видео
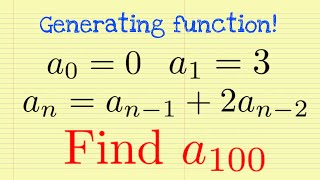
Generating function part 2 || recursive formula
Просмотров 4,4 тыс.4 года назад
Generating function is a powerful tool in discrete mathematics. Today, we are going over how to use generating function to derive closed form of a sequence given you know a recursive formula of it. Oh, and just in case you wonder, a100 = 2^100 1. Facebook: N-choose-k-RUclips-105387694184879/ Twitter: KuangSittipong Website: www.math.ucsd.edu/~sithamro/ Video information...
Generating function explained
Просмотров 19 тыс.4 года назад
Generating function is one of the most important, and yet, most confusing topics in discrete mathematics and combinatorics. Today, I am going over basic of basic of generating function, including addition and multiplication of generating function and examples. In the video at 6:49, you may notice and the constant term of the generating function and the value at n=0 are different and here is why...
Limit of quotient of sum || a problem from JEE advanced
Просмотров 4744 года назад
Today, we talk about a problem from 2019 JEE advanced. It involves computing limit of quotient of a finite sum. To solve this problem, Riemann sum would have to show up. Facebook: N-choose-k-RUclips-105387694184879/ Twitter: KuangSittipong Website: www.math.ucsd.edu/~sithamro/
Brief introduction to Stirling numbers
Просмотров 27 тыс.4 года назад
Stirling numbers are defined and used in Combinatorics and discrete mathematics. In this video, we go over definition of Stirling numbers of both kinds, describing what they counts (set partition and circular set partition), and their recursive formula. Facebook: N-choose-k-RUclips-105387694184879/ Twitter: nchoosekYT Website: www.math.ucsd.edu/~sithamro/ Video informat...
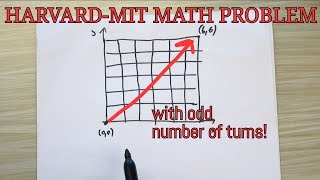
Harvard-MIT math problem solved easily
Просмотров 4864 года назад
What is the number of ways to walk from (0,0) to (6,6) on a grid so that you turn odd numbers of times. This problem was adapted from 2018 Harvard-MIT Mathematics tournament (HMMT), combinatorics round. This problem might seem hard and tedious, but with some insightful observation, the problem can be solved in two lines. Facebook: N-choose-k-RUclips-105387694184879/ Twitter: twitte...
How to compute this integral? || ln(sin(x))
Просмотров 6 тыс.4 года назад
Integration of ln(sin(x)) from 0 to pi/2. This integral was posted on twitter by professor Steven Strogatz. This involves so many must-know techniques like u-substitution and double angle formula. Original tweet: stevenstrogatz/status/1191789558507212800?s=20 Facebook: N-choose-k-RUclips-105387694184879/ Twitter: nchoosekYT Website: www.math.ucsd.edu/~sitham...
1, 2, 4, 8, ... || What's next?
Просмотров 11 тыс.5 лет назад
Find the next term of the sequence 1, 2, 4, 8, ?. The result might surprise you. In this video, we go over how to find a formula that satisfies any sequence of finite length. Facebook: N-choose-k-RUclips-105387694184879/ Twitter: nchoosekYT Website: www.math.ucsd.edu/~sithamro/ Video information: Shooting site: library.ucsd.edu/visit/study-spaces/view-all-study-rooms.html
12 ball puzzle || Complete analysis
Просмотров 5 тыс.5 лет назад
12 ball puzzle or 12 ball problem is a classic puzzle that asks you to identify the odd ball among 12 balls. The odd ball is slightly heavier or lighter than 11 other balls. You can use a balance scale 3 times, and you have to pick out the odd ball and specify if the odd ball is heavier or lighter. In this video, we completely breakdown 12 ball problem, so that you can solve this problem by you...
Monster problem with cute solution (Awww)
Просмотров 5995 лет назад
This infinite sum might look like it is insanely complicated. There are definitely several ways to compute this sum, but here I am introducing a slick way to solve it; using telescoping sum. Facebook: N-choose-k-RUclips-105387694184879/ Twitter: nchoosekYT Website: www.math.ucsd.edu/~sithamro/ Video information: Shooting site: library.ucsd.edu/visit/study-spaces/view-al...
What do I think of these viral math memes?
Просмотров 2455 лет назад
Proof of 2=1, or the fact that 0.999...=1, or the famous 60/5(7-5), all of these viral math problem or viral math meme has one thing in common. And I hate it so much. knowyourmeme.com/photos/221795-troll-science-troll-physics ruclips.net/video/wzchhbrqIBI/видео.html knowyourmeme.com/photos/55391-where-is-your-god-now themetapicture.com/what-is-the-correct-answer/ www.thecalculatorsite.com/artic...
3 hat puzzle || Can you explain what happened?
Просмотров 1,1 тыс.5 лет назад
3 hats, either red or blue, are given to 3 people. Then they must raised their hands if they see a red hat. First person who guess the color of his hat correctly wins. Everyone raised their hands. After a few minutes, one person shout 'red hat' and he won. What happened? This puzzle if fun to think about. You just need to think hard on what do you know, and what do other people know.... Faceboo...
What is n choose k? || formula, examples, Pascal's triangles
Просмотров 9 тыс.5 лет назад
N choose k, a nickname for binomial coefficient n,k, is one of the most important number in discrete mathematics. It is defined as the number of ways to choose k objects out of the collection of n objects. In this videos, we fully explain what n choose k is, formula of n choose k in term of fraction of factorials, 2 examples, and relationship to the famous Pascal's triangle. Facebook: facebook....
Mathematics behind shopping || How to shop smart!
Просмотров 9275 лет назад
Discount. Extra. Buy one get one. Buy one get one 50% off. All those deals can be confusing when you want to compare them. In this video, we break down most common deals and how to do calculations to help you make good decision when you go shopping. Source of memes: www.memedroid.com/memes/detail/732370 cheezburger.com/3820832000 knowyourmeme.com/photos/1324192-these-4-useless-things Famous RUc...
Prove mathematics identities by art and craft
Просмотров 1,1 тыс.5 лет назад
If you ever think that art & craft and mathematics have nothing in common, think again. In this video, I literally use art and craft to prove math identities, a^3-b^3 and a^3 b^3. Template can be found here: drive.google.com/file/d/1PFo2iV10odhfySZxSXV8AJtWvqr2s_EA/view?usp=sharing Facebook: N-choose-k-RUclips-105387694184879/ Twitter: nchoosekYT Website: www.math.ucsd....
2018 IMO problem 4 || This one is hard!
Просмотров 5485 лет назад
2018 IMO problem 4 || This one is hard!
Answer all questions on r/homework help
Просмотров 9 тыс.5 лет назад
Answer all questions on r/homework help
Who will win this game? || Revisit a math problem from middle school
Просмотров 3395 лет назад
Who will win this game? || Revisit a math problem from middle school
How to solve a remainder problem || Chinese Remainder Theorem Algorithm
Просмотров 3495 лет назад
How to solve a remainder problem || Chinese Remainder Theorem Algorithm
We solved a Harvard-MIT problem, and made it harder || Bonus question from 2019 HMMT
Просмотров 4935 лет назад
We solved a Harvard-MIT problem, and made it harder || Bonus question from 2019 HMMT
This problem is easy to understand, but how about solving it? || 2019 HMMT Combinatorics Problem 4
Просмотров 4,5 тыс.5 лет назад
This problem is easy to understand, but how about solving it? || 2019 HMMT Combinatorics Problem 4
Can I do high school math exam? Watch until the end for the result.
Просмотров 8745 лет назад
Can I do high school math exam? Watch until the end for the result.
How to survive playing this game: Josephus problem
Просмотров 1,7 тыс.5 лет назад
How to survive playing this game: Josephus problem
Dear blackpenredpen fans: How to cut a semicircle
Просмотров 1545 лет назад
Dear blackpenredpen fans: How to cut a semicircle
Pigeonhole Principle example: Why are there always a pair of relatively prime numbers.
Просмотров 3645 лет назад
Pigeonhole Principle example: Why are there always a pair of relatively prime numbers.
Pigeonhole Principle: How to apply step by step.
Просмотров 3765 лет назад
Pigeonhole Principle: How to apply step by step.
How to integrate ln(x)? It might not be as easy as you might think...
Просмотров 1345 лет назад
How to integrate ln(x)? It might not be as easy as you might think...
Debunk Integration by parts! Fully explained how to pick u and dv
Просмотров 895 лет назад
Debunk Integration by parts! Fully explained how to pick u and dv
Let's go over: Vector Calculus: Ep.5 Functions and Contour diagrams (Level curves)
Просмотров 955 лет назад
Let's go over: Vector Calculus: Ep.5 Functions and Contour diagrams (Level curves)







clean
I was looking for this solution for so long!
Using binary math, where "0" is blue, and "1" is red. 001 - 3 versions (001, 010, 100) 011 - 3 vers 000 - 1 vers 111 - 1 vers Assume all versions (ie: 001, 010, 100) amount to the same thing, so just handle the once case shown (ie: 001). So, for 011, like in the video, if you are the middle "1", and the right "1" raises their hand, and you see the left "0", then you know you are "1" (red). Also, for 001, if you are the middle "0", and the right "1" doesnt raise their hand, then you know you are "0" (blue). For 000, if no one raises their hand, you know you are "0" (blue). For 111, everyone raises their hand. According to the video, if no one solved it as above, after a few minutes, then you are "1" (red).
Johny Carson once played this game, although I am not sure they got the answer: ruclips.net/video/PKTk4coq05U/видео.html
However, I think someone in the Johny Carson comments gave the best non-math answer (I edited the colors here from black/red to red/blue to match with the above video): "I initially had trouble understanding it, so this seemed a good way to explain: If all three raise their hands, indicating they see at least one red hat, we know the possibilities are limited to there being either 0 or 1 blue hats because if there were more, at least one person would not raise their hand. Suppose the ultimately successful applicant is indeed wearing a blue hat. It would be as if it were emblazoned with fluorescent letters: “This the ONLY blue hat!” If the others saw such a hat, they would know their own hats were red and shout out the answer. Since this did not occur, the successful applicant concludes he’s not wearing a blue hat, so his must be red. This of course assumes the other two are smart enough to realize there can be no more than one blue hat, but NOT smart enough to play the same waiting game as he does. If they all used the same tactic, they would each assume they had a red hat…and be right."
In other words, 000, no one raises hands, 001, one person does not raise their hand, and 011 all people raise their hands. Since all people raise their hands, you assume its either 011 or 111. If it were 011, someone not wearing the blue hat ("0") would see the blue hat and know that the only scenario where there is a blue hat and all people raise their hands is 011, then immediately conclude they have a red hat. But since this doesnt happen right away, for smart people, it means if all people raise their hand, it must be 111, and they conclude they have a red hat.
Thank you. I lost sleep over this one last night.
But if you just put 1 in the first or the second box, the box that is left will be empty too? Could you elaborate on that?
each box must have an element according to definition of the problem
helll yeah
@ 10:47 I would have used the substitution u = pi - x instead.
@ 5:28 You didn’t close the bracket
Helped me so much dude, thanks! Keep up the good work :)
Thank you, this is an excellent explanation
why couldnt the same logic be applied in saying "we just have to find 4 ways to place b"? Man this is STILL confusing. Im sorry but for me it's always been true; this the one branch of maths i hate the most. And i sooo want to love it, like you do. gods.
Thank youu
thank you that was helpful
thx a lot dude
This video was an absolute lifesaver for my exams, thank you so much!!
Glad someone actually took the time to explain the intuition behind and not just fucking algebra
In easy problems it's just a logic, but in classical algebraic problems you need to learn advanced theories first.
would love to see more about stirling numbers, it'll be fun to see them in their generating function's context, and the thing you talked about linear algebra
That is sooo cooool
1★★★★★★
Thank you
It's actually 7 then 5 then back to 1 then it loops to infinity no matter how high the number gets. It's also how everything is made. Even you. 1+1 = mum and Dad 2+2 = grandma and Grandad and so on and so on it goes
Not only there is a recursive formula but also an explicit formula.
This was super helpful! I was trying to figure out this problem earlier (from another source), and even when looking at the solution I could follow it, but not figure out how to solve it myself systematically. Your method of looking at # outcomes vs # answers was super helpful, and let me understand the problem a lot better :)
I did it in a different way ruclips.net/video/XAlADhAgYAQ/видео.html
thank you so much this was so clear and really helped me understand it!!!
It was very helpful for me. Thank you.
Unfortunately, the quality is horrible, but the content is really interesting ...
great
@5:36, for instance, the coefficient for x^18 can be extracted from Wolfram Alpha as: SeriesCoefficient[3x/(1-x-2x^2),{x,0,18}]
Excellent presentation! You are the anti-math.SE in tone and friendliness!
Cool
4:33 shouldnt the no of items of arrangement be 5p3?
This is an excellent explanation, I could feel the lightbulbs going off in my head every ten seconds.
Thank you. I am so glad you find the video helpful to you!!
16 is next
Oh man This is way longer than expected
Fantastic explanation! Thank you so much for the effort. Really appreciate it
Glad it was helpful!
I like this guy's attitude.
I like you too!
Where you from
Thailand bro!
it was a good knowledge to get..upload videos like this pls.
Will try!
Good 👍👍
Thank you! Cheers!
Another cool, general way solving recursive relations is using matrices: *r_n := (x_{n-1}, x_n)^T ∈ ℝ^2 => r_n = A * r_{n-1}* with *A = (0; 1// 2; 1)* and the initial condition *r_1 = (0; 3)^T* . Via induction we get *r_n = A^{n-1} * r_1* though we need _Jordan's Matrix Transform_ to simplify the matrix exponential: *A = T * D * T^{-1}, | D = diag(2; -1), T = (1; -1// 2; 1)*
Awesome!
That was really good!
Can you do a video of THE EXTENDED BINOMIAL THEOREM with generating functions
Oh, I have been wanting to do this! Thanks for the suggestion!
Thank you, this was very helpful.
Glad it was helpful!
Great video; thanks!! So never seem to want to solve X, do we
i feel like one can also use this n choose k to practise magic
Thank you for this video dude! Appreciate your attention to detail in explaining the concept. Much help in solving my Combinatorics hw haha
the final problem is unexpected, but it helped me, many thanks to you!