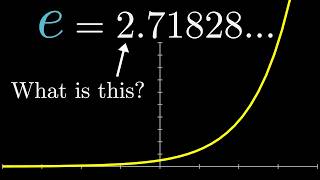
A Nice Exponential Equation (e^x=x^e)
HTML-код
- Опубликовано: 22 дек 2024
- Join this channel to get access to perks:→ bit.ly/3cBgfR1
My merch → teespring.com/...
Follow me → / sybermath
Subscribe → www.youtube.co...
Suggest → forms.gle/A5bG...
If you need to post a picture of your solution or idea:
in...
#ChallengingMathProblems #ExponentialEquations
EXPLORE 😎:
Simplifying a Complicated Algebraic Expression: • Simplifying a Complica...
Solving a Polynomial System in Two Ways: • Solving a Polynomial S...
A Trigonometry Challenge: • A Trigonometry Challenge
PLAYLISTS 🎵 :
Number Theory Problems: • Number Theory Problems
Challenging Math Problems: • Challenging Math Problems
Trigonometry Problems: • Trigonometry Problems
Diophantine Equations and Systems: • Diophantine Equations ...
Calculus: • Calculus







x=e omg Im so genius
Bro, how did you figure that out it must be so hard... I could never 😮
Big brain
Harvard, give this dude a scholarship
same, I think it is when I see the question lol
No korean
You could take second derivative to show that f''(x) > 0, and hence x=e is a global (and the only) minima. Hence x=e is the on ki y solution.
"minima" is plural
This is only true for continuous functions though, so you have to be careful with such analysis. It doesn't apply to x
@@UltraLuigi2401 doesnt matter… his idea was conveyed
@@HenrikMyrhaug Definitely good to keep in mind, though the video does restrict the domain to x>0.
How does f"(x) > 0 show it is the only minimum? Doesn't it just show the fluctuations locally
The easiest way is by inspection. Can instantly see x=e is a solution, and the graphs of e^x and x^e are well known, monotonically increasing, and only cross at one point. QED
But if that still is not enough to convince you, just manipulate the equation to get all the x's on one side
e^x = x^e
x*ln(e) = e*ln(x)
ln(e) / e= ln(x) / x
From which it is obvious that the only real solution is x=e
How do you reach QED from the information in the first sentence? Two curves being monotonically increasing can have more than one intersection.
@@diploma2007 You seem to have missed "well known". Those two curves only have one point of intersection. They are well known functions with similar shapes. And for those who are not familiar with those well-known functions, I included an additional argument.
@@XJWill1 if you mean 'well known' as in functions of the form x^C and C^x. I don't make it in general that these have only one intersection. For example x^2=2^x has intersections at x=2 and x=4
@@diploma2007 Good example. I concede the point. For x^c = c^x and except for c = e, every other real c > 1 results in two solutions. Only with c = e is there one solution.
@@XJWill1 yea but wouldnt most people replace ln e with 1 and remove that term from the eqjatio and and therefore miss that fact?
and there are a lot more complex solutions of the kind e^(-W_k(-1/e)) where W_k is the k-th branch of the Lambert W function, that is the inverse function of this function: f(z) = z e^z. (So, for example: since 1*e ^ 1 = e; then 1 is W_0 (e) (there are many complex branches, 0 returns the real answer, when there is one))
(for those wondering:
e^x = x^e
x = elnx
x/lnx = e
lnx/x = 1/e
-lnx * x^(-1) = -1/e
(-lnx)*e^(-lnx) = -1/e
-lnx = W(-1/e)
x = e^(-W(-1/e))
)
That’s sexy, and I had my hopes up this video would go that direction.
We can have another short-cut and simple solution. Raise both sides to the power (1/xe) so that we get e^(1/e) = x^(1/x). And the result follows by comparison i.e., x = e.
Good thinking!
But x^(1/x) is not bijective. You would need to take its derivative at e anyway
What do you mean by "follows by comparison"?
@@frenchimp For example, if x^(1/x) = 5^(1/5), one of the values of x is 5.
x=e as a valid solution is pretty much given from the get-go, since e^e = e^e, as mentioned in the video. But neither approach definitely proves that there are no other solutions for the problem, only that the trivial one definitely is one.
I am procrastinating doing my math homework by watching this. You explained this in a proper amount of detail, enjoyed it.
Another way to solve this problem is the following:
let's x=e^(t+1) so that e^(e^(t+1))=e^(e*(t+1)).
This implies that e^t=t+1, which is true only for t=0, as we can see if we write the maclaurin expansion of e^t.
We can conclude then, that the only solution to the problem is x=e^(0+1)=e.
After checking x > 0, just substitute x = e^(lnx) on the right side, and then we get e^x = e^(x*lnx) -> x = x*lnx since e^x is increasing and therefore one to one => lnx = 1 => x = e
e^x>0 doesn't imply that x>0
@@josephmathews6096 x^e > 0 implies x>0. If x were negative then x^e would be a complex number and it’s not even uniquely defined.
My approach was to differentiate both sides with respect of x, so the equation e^x = x^e becomes e^x = ex^(e-1). This means that x^e = ex^(e-1). [since the LH side remains unchanged when differentiated, the two RH sides are equal]. Divide both sides of this equation by x^(e-1), to get x = e. [Incidentally, by inspection, x 0, otherwise the original equation becomes 1 = 0. Further x cannot be negative, otherwise the original equation will always have a positive LH and a negative RH]
Same
Actually, if x is negative, the right hand side would be complex, as e is not an integer.
When you are looking for a value for x, you can't differentiate, since you would get 1=2 from something like x=2x+1
Awfully wrong reasoning. The zeroes of f have nothing to do with those of f'.
That tells you where two functions have the same derivative. But the original question is to find where the two functions intersect. You can have two functions intersect at a point where they have different slopes!
You can plot the graph of y=x and y=e.lnx and find the bisect points.
I dont think you can accurately plot e*lnx, and going this way you could plot e^x and x^e in the first place too
The two curves osculate at (x, e).
@@madghostek3026 (sorry about english)
I took the derivative of elnx, we know that it is equal to x. So I set the derivative = 1 and find the root. After that I placed the root in elnx to find the point which y=elnx is parallel to y=x. You can see how many roots are there by checking if the point is over or under or on the function y=x.
At the fork, x = eln(x), you have e^-1 = ln(x)/x = x^-1 * ln(x) = ln(x) * exp(ln(x^-1) or rewriting:
e^-1 = ln(x) * exp(ln(x^-1) multiply both sides by -1 and we have
-e^-1 = ln(x^-1)*exp(ln(x^-1) now take the Lambert w function of both sides and you have
-1= ln(x^-1) or exp(-1) = x^-1 or 1/e = 1/x and x = e
In the entrance exams of Japanese university, this is one of typical questions. For example, solve 2^x=x^2. In short, there are many question using the function, lnx/x.
I've seen harder problems on those entrance exams in a math journal but that was a while ago. I'll try to share if I find them. Do you have a link to their website?
@@SyberMath As I’m Japanese, I have difficulty finding the problems written in English, but I know the problems “Which is the larger e^π or π^e” has often been given in the entrance exams.
Magic math Trick 👉👉
ruclips.net/user/shortsuyPllWuSyuw?feature=share
@@playingmathswithparul8141 THE BALANCED, ULTIMATE, TOP DOWN, AND CLEAR MATHEMATICAL PROOF REGARDING THE FACT THAT E=MC2 IS F=MA:
Time dilation ultimately proves ON BALANCE that E=mc2 IS F=ma, AS ELECTROMAGNETISM/ENERGY IS GRAVITY. Time is NECESSARILY possible/potential AND actual IN BALANCE, AS E=MC2 IS F=ma; AS ELECTROMAGNETISM/energy is gravity ON BALANCE. Gravity is ELECTROMAGNETISM/energy.
Great !!!! QUANTUM GRAVITY !!!! E=MC2 IS F=ma. This NECESSARILY represents, INVOLVES, AND DESCRIBES what is possible/potential AND actual IN BALANCE. What are the EARTH/ground AND the SUN are CLEARLY E=MC2 AND F=ma IN BALANCE. Very importantly, outer "space" involves full inertia; AND it is fully invisible AND black. The stars AND PLANETS are POINTS in the night sky. GRAVITATIONAL force/ENERGY IS proportional to (or BALANCED with/as) inertia/INERTIAL RESISTANCE, AS E=MC2 IS F=ma; AS ELECTROMAGNETISM/energy is gravity. Gravity/acceleration involves BALANCED inertia/INERTIAL RESISTANCE, AS E=MC2 IS F=ma; AS ELECTROMAGNETISM/energy is gravity. BALANCE AND completeness go hand in hand. It does ALL CLEARLY make perfect sense. GOT IT !!!! THE SKY is BLUE, AND THE EARTH is ALSO BLUE. Great !!! Now, think about the man who IS standing on what is THE EARTH/ground. Perfect !!!!
Energy has/involves GRAVITY, AND ENERGY has/involves inertia/INERTIAL RESISTANCE. "Mass"/ENERGY IS GRAVITY. ELECTROMAGNETISM/energy is gravity. E=MC2 IS F=ma ON BALANCE. Gravity/acceleration involves BALANCED inertia/INERTIAL RESISTANCE, AS E=MC2 IS F=ma ON BALANCE; AS ELECTROMAGNETISM/ENERGY IS GRAVITY. (ACCORDINGLY, the rotation of WHAT IS THE MOON matches it's revolution !!!) Gravity IS ELECTROMAGNETISM/energy. TIME DILATION ultimately proves ON BALANCE that E=MC2 IS F=ma, AS ELECTROMAGNETISM/energy is gravity. News flash ! Gravity IS ELECTROMAGNETISM/energy, AS E=MC2 IS F=MA. The Earth (A PLANET) is a MIDDLE DISTANCE form that is in BALANCED relation to the Sun AND the speed of light (c), AS the stars AND PLANETS are POINTS in the night sky; AS E=mc2 IS F=ma. TIME is NECESSARILY possible/potential AND actual IN BALANCE, AS E=MC2 IS F=ma; AS ELECTROMAGNETISM/ENERGY IS GRAVITY !!!
By Frank DiMeglio
@@catalina7200 That is very easy ,
The language looks lengthy but mathematical solution is way too short.
1st Assume any of these as a greater one.
2 Take log both sides taking π or e as base.
3. Since π and e are positive numbers , therefore we can multiply them in inequality too.
4. Do some easy manipulation and the result will come automatically.
5. If your assumption comes right then you are right , if it comes wrong then answer will be opposite of your assumption.
Precautions -
Don't change the inequality sign like if you used (
Tried to do it in my head with purely algebra, great exercise
Thank you!
Awww, he drew the graph by hand. I was looking forward to seeing the Desmos graph in the document over the next week...
( :
😆
@@SyberMath me too
I followed the original equation until x/lnx = e; so x = e^a and lnx = e^(a-1). So, x = e^(e^(a-1)) = e^a; e^(a-1) = a; e^a/e = a; ae = e^a. Because of how linear and exponential functions grow, a = 1 is the only solution, therefore x = e^1 = e.
There are complex solutions as well (according to Wolfram alpha)
Fun fact. 2 to the 4th power is the same as 4 to the 2nd power. That fits perfectly in this equation.
The e in this is Euler’s number, it’s already defined
@@sauce8767 However, this appears to be the only example I can think of as 3 to the 9th is slot larger than 9 to the 3rd
6+9+6x9= 69
@@JosiahFickinger e isnt a variabl, its a constant equal to about 2.718
@@aryanjoshi3342 I'm sorry. I'm way behind in math topics because of my school experience, I never got the opportunity
Easier way to solve is that domain is x > 0.
We also know that if 0 < x 1
so we get 1 < x
We know that x = e is a trivial solution.
we also know that x^e < e^x when 0
The trick here is to raise both sides to the i2π power, x=e drops out immediately.
I feel like by using the fact that x=e gives an absolute minimum on the domain, we did not need to find the limits of infinity on both sides. Everything else > 0 => solution is unique.
that's right!
Magic math Trick 👉👉
ruclips.net/user/shortsuyPllWuSyuw?feature=share
0:38. e^x > 0 doesn't imply tha x > 0. x > 0 bc function x^e is defined at non-negative values of x
Differentiate both sides with respect to x. The left hand side exponential remains invariant after differentiation with respect to x. Equate both right hand sides and solve for x.
Everyone here doing math... just substitute the x with an e from the start... e^e=e^e
There's apparently 2 other solutions but they're complex, I don't know if we can calculate their exact values
How do you imply from e^x > 0 that x>0? I would say that x is positive because of the x^e is a power with real base and exponent.
I think you ignore the situation in complex number field. There can be countable infinite pairs of conjugate complex roots.
go to desmos and enter the formula y^x=x^y. You get two lines, one being y=x {x>0}, obviously, but also one that looks similar to y=1/x (it is definitely not this line, . Those two lines intersect at exactly (e,e). Every equation that has the form in the video will have two answers, one for each line, except when y or x equals e. You always have the simple answer of y=x, and the complicated answer (except in the case where y = 2 or 4, the only two points with integer solutions) that takes some work to figure out
The solution x=e is easy to spot, the hard part of the question is: Is it the only solution? We know that for all n in the natural numbers
exp(-x)*x^n -->0 as x--> infinity.
define f(x)=e^x-x^e and differentiate to obtain:
f'(x)=e^x-e*x^(e-1)
at the value, x=e, this becomes f'(e)=0. So for x>e, the exponential is growing faster than the polynomial, and there are no solutions for x>e. For 0
It's the only possible *real* solution, there are two more solutions in the complex world using the Lambert W function
a simpler solution (for me at least, since that's what I thought) passing x^e=e^e to Logarithms, which would result in ln(x)=x/e, and making the graph of both we can see that the only time they touch is in e
So, x=e
Isn't this obvious that for 0 < x < e, f' < 0 and for x > e, f' > 0 ? Also, the solution is the intersection of the line y = x, and the logarithmic curve y = e ln(x) which can have only one intersection.
That was a lot of work for an obvious solution.
You can't prove that there is only one solution
That's the point
Wolfram Alpha shows some complex solutions. Understandable, given e both as base and exponent.
Putting ln on both side then we have x=e ln(x) and differentiating both side w.r.t x we have dx/dx= e.d(lnx)/dx which implies x=e
u and other math youtubers are squeezing the life out of e , phi and i 😂
How about omega?
You mean blackpenredpen,mathologer,
Dr peyam, Micheal Penn,
3blue 1 brown
😂
I started similarly, but didnt use any calculus and got it using pretty much only log rules and equivalence.
e^(x) = x^(e)
xln(e) = eln(x)
x/ln(x) = e
x^(-1)ln(x) = e^(-1)
ln(x^(x^(-1))) = e^(-1)
log_e(x^(x^(-1))) = e^(-1)
since log_b(x)=a can be written as b^(a) = x, I can do this:
log_e(x^(x^(-1))) = e^(-1)
e^(e^(-1)) = x^(x^(-1))
this can be written as
e^(1/e) = x^(1/x)
its pretty clear that e is a solution which if I looked closer at the problem before I started it would have been pretty obvious from the get go.
From the step x=e.lnx, we can differentiate on both sides wrt x to get 1=e(1/x) -> x=e
That tells you that the functions have the same derivative at x=e. But that's not enough, on its own, to conclude that that's the only point where the original functions intersect. (What if they intersect at some other point where the derivatives are different?)
I have a question for you. Is (-5)^1.4 equal to (5/__180 degrees)^1.4 equal to 5^1.4/__180*1.4 degrees? "/__" is a phasor angle symbol representation.
Finally, something actually really interesting with this type of equations instead of just introducing the Lambert function once again.
Nice video
Glad you enjoyed it!
@@SyberMath be my maths teacher
Magic math Trick 👉🤔
ruclips.net/user/shortsuyPllWuSyuw?feature=share
e^x = x^e
just differentiate both side w.r.t x
e^x = e × x^(e-1)
=> e^(x-1) = x^(e-1)
now divide this eqⁿ with the original eqⁿ
e^(x-1-x) = x^(e-1-e)
=> e^(-1) = x^(-1)
here the powers are same so bases can be equated so e = x
The value ‘e’ in e^x is rational, because it’s derivative is always the same: e^x. Non-obviously, you’ve given the equation for a magnetic field: Irr.^Rational = Rational^Irr. which is a differential equation that can only be simplified in a finite system. One answer is “x is an electron in the base, and a proton in the exponent.”
you can just do this..
e^x = x^e, raise both powers with 1/xe
x^1/x = e^1/e
rewrite as (1/x)^-1/x = (1/e)^-1/e, multiply both powers with -1, we get (1/x)^1/x = (1/e)^1/e , use super square root * ssrt (x^x) = x *
we get, 1/x = 1/e, so x = e
Hi, at 0:41 why does e^x > 0 imply that x > 0? e.g. e^-2 > 0, so e^x > 0 when x < 0 also. Many thanks.
because e, as a constant, is more than zero so any power of e is also more than 0. except if you take the limit of x as it approaches -∞, where it would equal 0
x = -e * W(-1/e)
Just one real solution, but infinity complex solutions
isnt it e^-W(-1/e)?
@@rewardkhaled6359 I don't think so, I tried again now, and I get the same result. Maybe I'm wrong, could you show your passages?
Good video, it is a fine solution and interpretation. But, why you didn't take solutions at the complex? Maybe great things happen there.
Good point
Thank you ...but why you don't use Lambert W function....actually it gives different answer ...I appreciate if you comment about that
Magic math Trick 👉👉
ruclips.net/user/shortsuyPllWuSyuw?feature=share
Given that x > 0 and exp(x) = x^e, we have that x = e·ln(x), implying that ln(x)/x = 1/e, which is equivalent to -ln(x)/x = -1/e. -ln(x)/x = ln(1/x)/x = 1/x·ln(1/x) = ln(1/x)·exp[ln(1/x)] = -1/e. Let y = ln(1/x), hence y·exp(y) = -1/e. The minimum value of y·exp(y) is -1/e, because the nth derivative of y·exp(y) with respect to y is given by (y + n)·exp(y), which is 0 for n = 1, y = -1, and is always positive for n > 1, y = -1, and for y = -1, y·exp(y) = -1/e. This implies that y·exp(y) = -1/e iff y = -1. Thus -1 = ln(1/x), implying 1/e = 1/x, equivalent to x = e.
you explained this very well, thanks for sharing
My pleasure!
This video in not actually solving an equation. It's just an excellent demonstration of how function analysis is done!
Thank you!
You should precise we're solving for x real (although it's kind of obvious) otherwise there are actually complex solutions using the Lambert W function. Nice video anyway but there are some mistakes like the fact that e^x > 0 doesn't imply that x > 0.
take logs to get x = elnx then differentiate using product rule. 1 = 0 + e 1/x hence x = e
You could skip all of that and just by inspection x = e.
it's so easy to find x=e,but i can't get the solution this is the only answer.
This video was very valuable for me.
Nice but, I think that this solution could be simpler. Notice that we are looking for one solution of x. The task can be solved visually, left and right should look the same.
2^x = x^2 x=2 3^x=x^3 x=3, ..... e^x=x^e x=e, and whatever you put into e. It is very good interview question:) Thank you:)
When you know that f(x0) = f'(x0) = 0 and that a:a 0, then you know that x0 is the only root.
f'(x0) = 0 and that a:a 0 implies that f(x) is decreasing heading from x=a towards x=x0 and then increasing heading towards x=b. This means there is no other xi such that f(xi)=f(x0).
When you also know that f(x0) = 0 you then know that there is no other root.
What about imaginary and complex solutions?
df(x)/dxe and >1 for all x
Kindly help me solve and understand this integral equation
Integral of root of 1- x^2 (dx)
This inspired me to generalize this problem, basically solving x^k = k^x, with k > 0, k ≠ 1 (if k=1, then x^1=1^x or x=1, this is a special case. As for why I exclude zero, it's easy to see: x^0 = 1 ≠ 0^x = 0).
We can do the following steps: first, take x^k=k^x and divide the right hand side in both sides, giving us x^k/k^x = 1. We can rewrite that as x^k * k^(-x) = 1 or x^k * e^(-ln k^x)=1 or x^k * e^(-x ln k) = 0. So far, so good. We can take the k-th root on both sides (since we already established k>0) and we get x*e^(-x * 1/k * ln k) = 1. For the sake of brevity, let's note (ln k)/k = λ.
If we take y = -x * (ln k)/k (the exponent of the exponential function) and solve for x, we do this: ky = -x ln k k/ln k * y = -x x = -k / ln k * y = -1/λ * y. We can backsubstitute into the equation we established a paragraph earlier and it will become -1/λ*ye^y = 1 or ye^y = -λ. We can use the Lambert W function which is the inverse of f(x) = xe^x to get y = W(-λ). Using the relation between y and x, we get that y = W(-λ) = -x * λ, we get the final answer that x = -1/λ*W(-λ), alongside the trivial solution of x=k for k>0. There are multiple branches of the Lambert W function, the most common ones being W_0(x), where the cutoff of the graph happens at 0 and W_(-1)(x), where the cutoff happens at -1. The solutions you get by using different branches of the Lambert W function will satisfy this equation, but they will be different. It's more complicated than what I've described here, so if you want to know more, check the Wikipedia article.
For the problem in the video, λ=1/e (scroll up to the definition of lambda) and thus x=e if we use W_0 and for W_-1 we get two complex solutions, something like -0.63009 ± 0.481814i .
Nice!
Aint no way I am watching this in my spare time. What has college done to my brain?!?!?
Now make it hard and show us e^x = ax^e, where a is any positive constant. Desmos shows a must be greater than or equal to 1 for this to have a solution, and Wolfram Alpha uses the Lambert function to solve it. I tried "guess and check" a few times before turning to the internet for solutions, and did not come up with anything.
Very beautiful explanation 😍
Thanks a lot 😊🥰
What if there is a solution as x=1? I mean you can write it as e^1=1^e and of course it’s wrong. But what watch 1 as lne so e^lne=lne^e. At the left of the equal you can remember that a^log(a)b=b so e^lne=e and at the right of the equal sign e*lne=e so it’s an identity and lne is a solution.
3:17 "...and this implies x equals e. OK, great."
He sounds disappointed.
And I think taking logs of both sides of this equation makes it really easy
I love your video.A lot of thanks .God bless you , dear professor
Thank you! 😃
The obvious solution comes to mind almost immediately - If X=e, then voila - no fancy algebraic manipulation or calculators required same as 452^X=X^452 and so X=452 in this case
The hard part is to prove that this is the only solution
x = e, all done! YAY! Next video! 😁
X=e is the only solution because if we compare on same base, then we get ln x= 1 which yields solution x=e
e^x is 1-1 function and x^e is not but because e^x is 1-1 , those two fanctions have only one meeting spot if they have and x=e is obviously a roost so only for x=e we have this equation ,second way is to solve using ln function in both sides for x>0
Omg i cant solve it my wrong solution is 💀
We get x=e.lnx
Graphs meet at x=e from starting symmetry(if you have eyes you can see), if it is only solution check if y=x is tangent to y=e.lnx, y'= e/x at x=e (y=e) and y'=1 so y-e=1.(x-e) ==> y=x
So as y=x is tangent to y=e.lnx we have no other solution other than symmetrical x=e solution 😂
It’s e because that is the intersection of the line and curve that satisfy x^y=y^x. Another example of how e shows up literally everywhere in mathematics
Even though I haven’t taken calf yet, I understood everything perfectly
What about complex solutions?
Equation define on ℝ⁺.
x = e ln(x) => x/ln(x) = e (Because 1 is not solution)
quick and easy function study of x → x/ln(x)
There's only one possible solution: e.
Reciprocally e is a solution.
So eˣ = x^e x = e
Aply neperian logaritm both sides and then derivative and you´ve got the solution :) greeting from Venezuela
I'm hung up on 0:36
Okay I sleepy-typed a bunch of stuff but my problem comes down to the way you presented it.
e^x > 0 for all real numbers. That is not a reason to restrict to x > 0.
_Wanting x^e to make sense_ is the reason for that.
Could have been presented in a way that didn't make my sleepy brain coast on "wtf" mode for several minutes.
*Keep making great content.* Sorry so sleepy. I do think I have a point, though. A critical piece of logic was omitted and _this_ viewer was confused thenceforth.
I found a pattern. Any number to the fourth power is equal the that number 2 squared to the 2nd power. It's really not surprising, actually.
e = x is a quick intuitive solution proven very easily with simple methods. It's cool that you took a more complex path for the answer. I'm glad you proved it was the *only* solution, as that is much harder and makes for a much more interesting dive.
what if x=4 & e=2
where x>e
@@pronabchakrobortty9163 e does not equal to 2
@@khytron06 do u want to mean that e is constant? if yes then x=e
@@pronabchakrobortty9163 e is a constant. It is called Euler’s number
Set pi = 3
I feel it's more likely to get a task with this equation that reads "prove that e is the only possible value" rather than just solve
I have a question
If 0 = ax^1 + b has 1 solution
0 = ax^2 + bx + c has 2 solutions
Then ax^e + c has 'e' solutions??
I took both sides to power 1/(ex)
I get x=e
That's nice!
Can you solve for y:
dy/dx = e^y ???
Sure
y=-Ln(-x+k), k is a constant
Thanks for sharing! You made it look e-asy!
You are so welcome!
At 0:44 you say that because e > 0 and therefore e^x > 0 for all x that it follows that x > 0.
However I’m not sure that’s true. Replace e with 2.
2>0 and 2^x > 0 for all x. Nonetheless 2^x = x^2 clearly has a solution with x < 0
The thing is that 2 is rational and e is irrational. If we want a real answer for a negative number raised to a real power, we can only take rational powers (so 2 is OK but not e), and even then with restrictions. E.g. (-1)^(1/3)=-1, but (-1)^(1/2) is not defined (as a real number). The general rule is that a^(p/q) [with a0 we can extend the definition of a^x from rational to irrational powers x by continuity, but not for a
@@MichaelRothwell1 nice reply. Yes I guess if you use complex numbers you can establish that there’s no solution with x < 0 and real.
But I just don’t think the way it’s presented in this video is rigorous. Perhaps understandable.
@@nicks210684 I totally agree. The "solution" presented is clearly based on already knowing the answer, and really gives no clue as to how to go about solving this type of problem. I solved it by assuming (wrongly, as it turned out) that the answer was of the form a-b√6, where a & b are rational. This led to equations which I solved. The values I obtained were not rational (so my assumption was incorrect), but when substituted in, gave a value that worked, and simplified to 5√2-√3. Only then did I realise that a solution of the form a√2-b√3, where a & b are rational, was another possibility I should have considered. So I started again with this form, and this time it worked!
The result that there is no real solution for x 0 follows x > 0 is incorrect, because e^x > 0 is true for all x.
e^x > 0 does not imply that x > 0. For example, set x = - 2, and see that e ^ - 2 > 0. You don't use this except to exclude negative x. But you can exclude negative x by virtue of the logarithm, provided you are looking for real solutions.
That's right!
A bit strange logics: x is positive in this equation not because the exponent function is positive, but because of the constraints on the definition area of the function y=x^e. Since e is irrational, then some negative powered in e has no sense in real numbers.
Yes thanks ! It was triggering me, but I was not sure because I'm a little rusty in math. It didn't seem at all obvious that x^e > 0 => x > 0 with the given justification (which just say that e^x > 0 => the solution must respect the condition x^e > 0). In fact, that is totally wrong for any even power function (if we take 2 instead of e for instance). It is just that, as you said, x^a is not defined for x < 0 if a is not en integer. It is a prerequisite of the problem that, if x is a real number, then it have to be positive or equals to 0 (you can then get ride of the 0 case just by testing it).
x^e is defined only for positive x! Therefore, we do not need that e^x is positive for x>0
Use lambert function. The sum is easy. But if one is unknown of lambert function then possibly it will be impossible to solve.
So A^x = x^A has 2 solutions for A=2, 2 and 4. One solution A=e - seems for values A>1 there are 2 solutions - wonder what happens with A on (0,1)?
The Lambert W function makes the solution trivial.
Well done 👍
Thank you! 😊
Looks like one of those where you have to use LambertW.
A Japanese university's entrance exam asked us ''count the next equation's solution e^x-x^e=k (x>0) (k is constant)''.
Another great explanation, SyberMath!
There is easy solution, both side exponential by 1/X...And exponential by 1/e...you will find e^1/e equal to x^1/x ...which means x=e.
Me: Oh, it looks complicated.
Also me: Move this here, move that there... Oh, x=e !
Then I checked the video and this is right.
Nice solve! Thanks for making it!
No problem!
Is it just for e or any base.
What my mind says,
If a^b=b^a
Then it must satisfy only when a=b because 3²≠2³(for example) but 2²=2² or 3³=3³
The logic behind claiming x must be positive escapes me. If i had the equation e^x = x^2, there is only a negative solution against the logic being employed. I also don’t understand what x^e is for negative x.