The Stress Tensor and Traction Vector
HTML-код
- Опубликовано: 9 фев 2025
- This video is part of a series of videos on continuum mechanics (see playlist: • Continuum Mechanics .
Keywords: continuum mechanics, solid mechanics, fluid mechanics, partial differential equations, boundary value problems, linear elasticity, small strain elasticity, infinitesimal strain elasticity, Cauchy stress tensor, traction
Recommendations:Visualization of tensors - udiprod • Visualization of tenso...
Music:
Prelude 2 - VGM Mark H / prelude-2 Abandond Station - VGM Mark H / abandoned-station








Awesome video! Felt completly lost after my class on continuum mechanics where the professor just proved a bunch of formulas, but now it finally makes sense:)
Absolutely brilliant !!! The Strength of materials and Continuum mechanics classes should be teached like this. Rigor always come after intuition. First create some intuition then jump into the rigor. Which is rare among the lecturers. Thank you dear Dr. Please dont stop making this videos. Future engineers and scientist needs mentors like u. God bless you
Thank you so much for this very nice and motivating comment!
I demonstrated that a symmetric stress tensor implies the conservation of angular momentum in my continuum mechanics exam, but I truly understood the concept just now. Thank you for showing the visualization.
Thank you for the videos, very simple and high quality.
loving this series so far!
🙏
You deserve millions of subscribers. Take love brother 💖💗💞
🥰
You are my favorite YT channel !
Thank you ! :D
Hello Dr.Simulate, my motivation to learn tensors is to calculate multi-axis fatigue. The critical plane method requires engineers to pull out stress components from FEA and manipulate these stress components using local coordinates. Your courses online in tensor and continuum mechanics are very helpful for me to understand the basic concept of tensor and its stress components. Thank you for your instruction video!
Amazing work! These visualizations about continuum mechanics helps a lot to understand faster the abstract part of this theory! Thank you so much!!!!
🙏
keep up doing god's work.
Good visualization. Your presentation shows that continuum mechanics is a kind of beautiful art.
❤
3:59 This is incorrect. Wikipedia says the opposite.
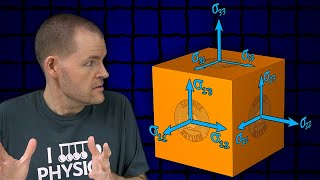
Assuming a material element (see figure at the top of the page) with planes perpendicular to the coordinate axes of a Cartesian coordinate system, the stress vectors associated with each of the element planes, i.e. T(e1), T(e2), and T(e3) can be decomposed into a normal component and two shear components, i.e. components in the direction of the three coordinate axes. For the particular case of a surface with normal unit vector oriented in the direction of the x1-axis, denote the normal stress by σ11, and the two shear stresses as σ12 and σ13.
NOT 11, 21, 31.
This comment was made several times. The index order does not matter for a symmetric tensor. It's just a matter of definition. I chose the index ordering the way I did, because in this way if you compute t = sigma * n with n being one of the unit normal vectors of the infinitesimal cube, you get the traction at the surfaces of the infinitesimal cube.
The formula t = sigma * n is commonly used in continuum mechanics. In wikipedia (en.wikipedia.org/wiki/Cauchy_stress_tensor) the traction is defined differently as T = n * sigma, which is why there the rows of sigma are the tractions acting on the infinitesimal cube.
@@DrSimulate Cool. The official index for all engineering purposes is i,j - orientation, direction. This is shown in practically all engineering textbook. For example, the xy shear stress is drawn on the x-face in all drawings of a 2D stress state. It is so confusing for students to see it flipped to j,i, randomly without explanation in your videos.
@@TheAncientColossus Yep, would be nice to mention it but I can only imagine how much there is to consider doing these videos so it's easy to overlook or forget.
@@johannesjoensuu7946 Fair point.
Nice video, thanks for the explanation, keep it up :)
Great video. Hope you make more videos.
Thanks!
thanks for your work
Beautiful video! Is there a reason why the indices of the stress tensor in your video are opposite to those of the Cauchy stress tensor? What I mean by that is I have been taught that the first index i indicates normal plane where stress is acting, and second index j indicates direction of the stress. Thanks!
@@SeanFlournoy Hey! I would argue like this: it is very common to associate the first index of a tensor/matrix with the rows and the second index with the columns of the tensor/matrix. This means that for example for the first column the second index is always one. Now take for example the vector n = (1,0,0), i.e. the normal vector of the right surface. Multiplying sigma with n to obtain the traction at this surface results in the first column of sigma (for which the second index is always one). This means that the second index defines at which surface the traction is acting.
Of course this discussion is a bit pointless for a symmetric tensor. It is always a matter of definition :)
I would like to argue in this sense that if you multiply the stress tensor with the vector n=[1;0;0], for example, you would obtain [sigma(11); sigma(21); sigma(31)]. So if you are multiplying the stress tensor with the vector [1;0;0], you are getting essentially a resultant traction vector in the 1 direction. The magnitude of "t" is the summation of all the cumulative forces in the 1-direction of the planes. The individual components of the "t" vector represent forces in 1-direction but in the different planes 1,2 and 3. So, in the "t" vector, the common index is the second index which should ideally represent direction. And the first index should represent the plane. Since the stress tensor is symmetric, it does not make a difference. If you tried doing the same with a non-symmetric matrix, may be it would make more sense what I am trying to convey here.
In the indicial notation, the equation is written as t(i) = sigma(ij) * n(j). So, if you consider the index "i" as the plane index and "j" as the direction index, then if you multiply the stress tensor with the normal vector, the result you obtain is t(i), where, i vaies from 1 to 3, you obtain [sigma(11);sigma(21);sigma(31)] which is numerically equivalent to [t(1);t(2);t(3)]. Since in the initial assumption, "i" is considerd as the plane index, this is folllowing the indicial notation., where sigma(11) ,sigma(21) and sigma(31) are the force vectors in the 1-direction but in the planes 1,2 and 3 respectively.
If I followed your assumption, the "i" would refer to direction and "j" would refer to plane. Even then, the equation would have to be represented as t(j) = sigma(ji) * n(i) [because sigma(ij) * n(j) is not equal to sigma(ij) * n(i)]. Even in this case, the [sigma(11);sigma(21);sigma(31)] would be equal to [t(1);t(2);t(3)] where "j" is the free index and has values from 1 to 3. Since "j" is the free index, and it was your own assumption to consider the "j" index as the plane index, it would mean that you are bound to consider the 1st index as the plane index to maintain the relationships of tensors.
So, it seems more consistent to use the conventional index notation, rather than the one you referred to in your video. I understand if you may disagree, but please correct me if I'm mistaken.
Your videos have been of immense help despite this confusion-thanks for the great content!
11:12 Why does the stress TENSOR increase on the narrow parts of the geometry? Or does the traction VECTOR increase?
Both should increase in the narrow part of the geometry. If you "cut" the geometry at any height, the resulting vertical force should be the same. In the narrow part of the geometry, the force distributes over a smaller area and hence the stress and traction are greater.
Thank you!!!
Thank you ❤
Amazing videos
Can you please advise me what kind of software that can be used to create the simulation in this video, Dr.? Many thanks.
Sure, I use Fenics for finite element simulations and then I use Manim and Matplotlib for the animations. :)
@DrSimulate thank you Dr
I thought traction was getting utilising friction to move, or something along those lines