Symmetric Groups Part 1
HTML-код
- Опубликовано: 10 фев 2025
- The symmetric group of a set is the set of all bijective maps from the set to itself.
This set of bijections along with the composition table defined by composition of the mappings satisfies the axioms of group theory.
In this video we specifically look at the finite symmetric groups, which are the symmetric groups of finite sets.
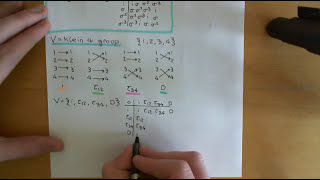
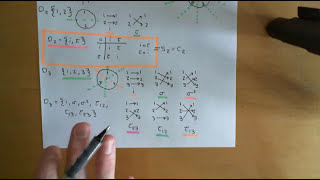
We look at the example of S3, which is the symmetric group of a set of three elements. This group has six separate elements. We look at what these six elements are and illustrate how to construct the composition table for this group.







Every subject should have an introductory course structured like this, instead of just pouring all the generalized concepts on the newbies... People need this and are searching for this, so it's a great work that you are doing, THANK YOU!
one of the most impresive things i cant really understand is how you have such a good handwriting, like wtf.👍👏
Yet another EXCELLENT exposition of Symmetric Groups. ..... Best on the "net". I'm going thru all your videos as part of my revision for exam on Group Theory in February 2020, so really grateful for all your instruction.
review ends at 9:30
wow thanks a lot
I was just thinking "when does this finish lol"
i enjoyed the review. did you see video 1,2,3?
2019 AND ME, just whatching these great viideos!
How can you know both Math AND Biology? That's unnatural :D
Do you have a Patreon? I'd like to donate...
Hi, I really enjoy your videos and they have been very helpful for me so far. Just wondering if you have any videos on the topic symmetric and alternating groups? So with stuff like the composition of disjoint cycle?
Beautiful sir ❤... Can you please tell one thing, that why is this group called symmetric???
You should make a playlist for analysis
i like it Elliot
9:36 - new topic starts
So if I am getting this right... The additive composition defined on decimal number system of S4={0,1,2,3} is not a group since when we do 2+3=5 we are losing the closure property.
But if we take the number system of base 4.. we get ourselves a Group. yes?
but it's not a group, just a set
Group operations modulo n. This works with all the regular number systems. Example:- consider Z as the set of all integers. Let( Z,*) be a group(It is, check!). Then Z(subscript5) is {0,1,2,3,4} closed under multiplication modulo 5
Defines natural numbers as number of cows. 1 cow, 2 cows, 3 cows.
and zero cow :)
Why must the identity element commute?
that's an axiom of Group
Suppose you have an i in your group and for any x in your group, x * i = x
for any a and b in your group
a * (i* b) = (a * i) * b because of associativity
a * (i * b) = a * b because of our previous assumption about i
Now you can see that putting i before be gives pretty much the same result as if nothing was put there when composing with other elements. We say that i * b = b to be able to directly simplify this kind of thing.
(those are just my thoughts, I'm no group expert)
Ook....?
You forgot zero in the natural numbers
Zero is not considered a natural/counting number historically as it was invented much later. Some authors do include it as a natural number, but it’s not universally so.
Michael Goldenberg thanks for pointing this out.