
- Видео 432
- Просмотров 284 467
Harare Maths
Зимбабве
Добавлен 25 сен 2009
Mathematics
Discrete Mathematics
Ordinary Differential Equations
Linear Algebra
Vector Calculus
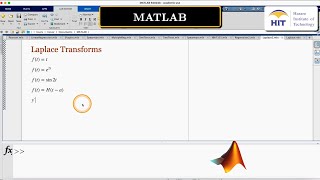
Laplace Transforms
Operations Research
Number Theory
Discrete Mathematics
Ordinary Differential Equations
Linear Algebra
Vector Calculus
Laplace Transforms
Operations Research
Number Theory
Видео
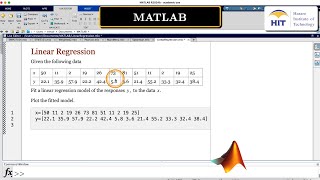
Matlab | Linear Regression | Ex 2
Просмотров 67Месяц назад
Matlab | Linear Regression | Ex 2 Link to excel file: docs.google.com/spreadsheets/d/1rzAYIdQ-ofqwZ-7PSo4FX-A8U96SKBV4/edit?usp=sharing&ouid=117534951395733811712&rtpof=true&sd=true
Matlab | Pearson's Correlation Coefficient | Ex 2
Просмотров 64Месяц назад
Matlab | Pearson's Correlation Coefficient | Ex 2
Matlab | Shapiro Wilk Test
Просмотров 132Месяц назад
Shapiro Wilk Test: testing for normality. Ahmed BenSaïda (2024). Shapiro-Wilk and Shapiro-Francia normality tests. (www.mathworks.com/matlabcentral/fileexchange/13964-shapiro-wilk-and-shapiro-francia-normality-tests), MATLAB Central File Exchange. Retrieved November 22, 2024.
Matlab | Pearson's Correlation Coefficient
Просмотров 121Месяц назад
Matlab | Pearson's Correlation Coefficient
Matlab | Standard Deviation | Standard Error
Просмотров 83Месяц назад
Matlab | Standard Deviation | Standard Error






Thank you, very well explained.
❤
Shouldn’t we use the least number with the most demand if it’s a tie
There are different ways of solving problems. The method you are suggesting and the one used here give the same solution.
you have really helped me understand this concept, the explanation was very straight foward,thankyou.
A good presentation of work. Keep it up fam
how to download on Mac??
Currently, the easiest way to install Maxima is using Homebrew. After installing that program, issue the following commands to install Maxima and Xmaxima: brew update brew install maxima brew install tcl-tk
wonderfull work Sir please keep it up
Thank you bro😊😊
Thanks very much sir
very good
very clear
Very understandable 😊
Proudly Zimbabwean🎉
Just WOW
Great
Thanks
Thank you sir❤
Great video
Wów, so amazing
Good
Decide which of the following integers are divisible by 22. (a) 0. Dr M for such a question if 0 is divisible by 22 how can we conclude is since the remainder is 0 0 is divisible by 22 ok?
Good evening sir for : Show that if a and b are positive integers and a | b, then a ≤ b. how would we express this or conclude this
Prove or disprove that if a | bc, where a, b and c are positive integers and a ̸= 0, then a | b or a | c. do we use counter examples for this one
i did :(Show that if a, b, c and d are integers with a and c nonzero, such that a | b and c | d, then ac | bd.) in two ways 1) where Given a∣b, we can write: 𝑏=a⋅k(1) Given c∣d, we can write: 𝑑=c⋅m(2) then using bd ,bd=(ak)(cm)=a⋅c⋅k⋅m. then we let x=km then we have bd=ac⋅x proving it but im not entirely sure about this approach, The second approach i took was a longer one where i said for ac | bd, bd=ac⋅z then from (1) and (2) i took the b and d equivalent (ak)⋅(cm)=(b/k)⋅(d/m)⋅z , this approach by substituting actually eliminated k and m leaving bd=ac⋅z
How about :Show that if a, b, c and d are integers with a and c nonzero, such that a | b and c | d, then ac | bd , I tried solving for the third by substituting the 1st and 2nd equations into it but the way its turning out im not sure how it would be showing that the statement is true
Sorry to bother this late, how about :Show that if a, b, c and d are integers with a and c nonzero, such that a | b and c | d, then ac | bd
I will try to post a tutorial on this when I get time. Or you can check from my office.
@@HarareMaths Thank you.
Good evening Dr M, Question 1 on the worksheet says :Does 18 divide each of these numbers? with emphasis to the word 'divide' would we use if a|b ∃c∈Z : b=ac as a solution. The reason i ask this , lets say for 1.c) 1005. of which 18 does not divide 1005 that i know so here divide would be the same as 18|b?
In number theory we write 18 divides b as 18 | b, then apply the definition of divisibility you mentioned.
@@HarareMaths Thank you
Very good. I appreciate your efforts
Dr M,do we have to rewrite the whole matrix or can we do just as you did by placing the brackets in the table
It’s better to rewrite the matrix, much clearer.
@@HarareMaths Thank you
Can you explain how you expanded it?
Nice
As an aside, if you are only looking for primitive roots, then it's often quicker to check the _largest_ factors of ϕ(m) first. Taking the example of m=13, we have ϕ(13) = 12. We know that r^12 ≡ 1 mod 13 (by Fermat's Little Theorem), so we don't need to check that. That means that r^6 must be congruent to 1 or -1 mod 13 (since r^6 is the square root of r^12). If r^6 ≡ 1, then r is not a primitive root. If r^6 ≡ -1 ≡ 12 mod 13, then we need to check r^4, but we don't need to check any further since none of r^1, r^2, r^3 can possibly be congruent to 1 if r^4 and r^6 are not.
Thanks for the explanation
❤❤❤❤
Is this maximization?
Minimization.
Very helpful 🙏
i wanted to ask if we are going to sketch the graphs on the test on Friday
Yes, the graphs are part of the solution.
Even 6 divides a³-a
Yeah
For checking a transitive relation in the form x>y do we use number examples such as above and if we do how would we use the number examples Can we use any combinations that can give us 15 and if lets say the numbers we choose are (8,7) for a>b its true but for b>c (7,8) is not true how would we tackle such a question saying x>y defines a relation on N determine if its reflexive, symmetric and transitive. On worksheet 2 .2b the question asks xy is a square of an integer determine the relations, how do we work it out
Have you looked at this video:Binary Relations ruclips.net/video/p8hJVz8HiaY/видео.html it looked at a relation defined by x>y
I see,thank you ,i have checked it out but what of xy is a square of an integer
What is the Criteria for symmetric nature in the first set, then transitive nature in the second set how is it obtained because (3,3) and (4,4) don't satisfy
(3,3) and (4,4) are not of the form (a,b) and (b,c) as stipulated in the definition
@@HarareMaths Oooh I see , thank you
I am in grade 10 and I only undrstood what is Integer,Natural no and odd no.😢