- Видео 286
- Просмотров 306 056
Learn Physics with Dr. Viv!
США
Добавлен 14 мар 2020
Physics and math at undergrad and graduate levels
GCM28: The linear Stark Effect using the Hamilton-Jacobi formalism
The time-independent Hamilton-Jacobi equation is separated in parabolic coordinates to solve the problem (in principle) of the motion of a non-relativistic electron in a Coulomb potential in the presence of a constant external electric field, the so-called Stark effect. The separability of the H-J equation in these coordinates was discovered by Jacobi, and the application to the linear Stark effect was first achieved by Karl Schwarzschild, of the Black Hole solution fame!
Просмотров: 428
Видео
GCM27: Projectile motion using the Hamilton-Jacobi Formalism
Просмотров 1 тыс.Год назад
After briefly introducing the ideas behind the powerful formalism arising from canonical transformations known as Hamilton-Jacobi theory, I apply the algorithm for solving separable systems laid out systematically to re-solve the rather trivial and well-known example of projectile motion in a constant gravity field and no air resistance. Examining this freshman physics problem in the most advan...
GCM26: Solving the Anharmonic Oscillator using Canonical Transformations
Просмотров 357Год назад
I introduce the important phase space transformations known as canonical transformations (or, amongst mathematicians, symplectomorphisms). These CTs have special properties: they leave the new action invariant, and the form of Hamilton's equations, with respect to a new Hamiltonian (known as the Kamiltonian) is unchanged. We show that CTs form a group, and talk about the Poisson Bracket formula...
GCM25: Noether's theorem, Noetherian charge, & conserved quantities
Просмотров 1,6 тыс.Год назад
We begin gently by discussing the connection between symmetry of time, space translations, and rotations and the corresponding conservation of energy, linear momentum, and angular momentum, respectively. Then we kick into high gear, and discuss the set of all Lie group transformations that leave invariant a certain modified (by exact time differential) action functional invariant, to the full v...
GCM24: Euler angles and precession of a heavy, symmetric top
Просмотров 6 тыс.Год назад
Euler angles to describe pivot-fixed rigid body motion are introduced. The top's Lagrangian is written out, conserved quantities found, and analysis is done in terms of the effective potential. We examine two limiting cases, of fast precession and slow precession.
GCM23: Euler equations: Spin stabilization, precession, & dinosaur extinction pole-wandering!
Просмотров 4502 года назад
I set up the Euler equations, and demonstrate how spin stabilizes wobble in a rotating system's flight. The equation relating precession angular velocity to the principal body angular velocity is derived to explain features of a gyroscope. As a fun example, I also estimate the extent to which the N pole of the Earth may have wandered on account of the impact from the asteroid that is credited t...
GCM22: Chassel's theorem and the moment of inertia tensor
Просмотров 3492 года назад
The important theorem that the total kinetic energy of a rigid body is a sum of its translational and rotational is proven. The moment of inertia tensor that results in the proof is then exemplified with two calculations: that of a cube and a laminar square plate. The principal axes are found for the latter example and interpreted geometrically. Erratum: in the 28 minute mark, when I evaluate t...
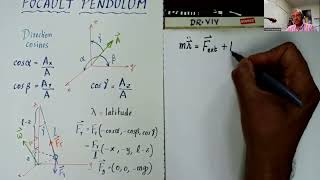
GCM21: The Focault Pendulum
Просмотров 2622 года назад
Here, we set up and solve the problem of the precession of the plane of a pendulum's oscillation due to Earth's rotation, using the equations for motion in a noninertial reference frame.
GCM20: Coriolis v. Newton
Просмотров 2372 года назад
The eastward deflection of a particle dropped from a height is derived after first deriving the equations of a noninertial reference frame: this is the computation of Coriolis. Then, we repeat the calculation but using the point of view that a particle dropped from a height is actually describing an elliptical orbit according to the laws of orbital mechanics. The two computations are shown to c...
GCM19: The Laplace-Runge-Lenz vector
Просмотров 8992 года назад
Additional symmetries of the Kepler problem are described and the novel geometrical interpretations as they relate to orbital mechanics discussed. A little bit at the end about group theoretical aspects of the problem.
GCM18: The Kepler Problem
Просмотров 2,2 тыс.2 года назад
The three laws of Kepler are proved for the case of an elliptic orbit under a central force, and for the specific case of attractive gravity.
GCM17: Gravity tunnel between New York City and Los Angeles
Просмотров 3032 года назад
The shortest transit tunnel is shown to have the shape of a hypocycloid and the transit time calculated, using the methods of the Calculus of Variations.
GCM16: The Brachistochrone problem
Просмотров 1 тыс.2 года назад
We solve the classic problem of finding the shape of the path in a constant gravitational field which minimizes travel time. First the elegant solution of John Bernoulli is presented, then the more technical solution using variational calculus and a specific corollary to the Euler's equation is presented. In closing, we discuss properties of the solution.
GCM15: Variational Calculus---prove straight line is the shortest path between two points
Просмотров 5862 года назад
The Euler equations are used to extremize the arc length functional to obtain the extremal curves, which are straight lines between two points on the plane. Next, we use the technique of first variation to perturb the optimum path and show that the obtained path is in fact a global minimum.
GCM14: Derivation of Euler-Lagrange's equations and Hamilton's equations
Просмотров 8102 года назад
Lagrange's celebrated derivation of "something from nothing" is shown, starting from D'Alembert's virtual work principle; then, using calculus of variations, we repeat the derivation using Fermat's principle and Hamilton's principle. Finally, a two vector variation done on phase space produces Hamilton's equations. The video concludes with a proof of Hilbert's bump function lemma, widely used i...
GCM13: Generalized forces and the Electromagnetic Lagrangian
Просмотров 7772 года назад
GCM13: Generalized forces and the Electromagnetic Lagrangian
GCM12: Free motion on a cone---existence and stability of orbits
Просмотров 8052 года назад
GCM12: Free motion on a cone existence and stability of orbits
GCM11: A nontrivial Legendre transformation---Atwoods machine with three masses and a spring
Просмотров 2082 года назад
GCM11: A nontrivial Legendre transformation Atwoods machine with three masses and a spring
GCM10: Ball rolling down a wedge that is NOT fixed!
Просмотров 1,4 тыс.2 года назад
GCM10: Ball rolling down a wedge that is NOT fixed!
GCM09: Double pendulum with air resistance
Просмотров 5932 года назад
GCM09: Double pendulum with air resistance
GCM08: Bead sliding down a smooth spiral---constraint forces
Просмотров 6752 года назад
GCM08: Bead sliding down a smooth spiral constraint forces
GCM07: Semi-Atwood's machine solved using SIX methods
Просмотров 2692 года назад
GCM07: Semi-Atwood's machine solved using SIX methods
GCM06: Throwing a ball vertically under quadratic drag
Просмотров 1,2 тыс.2 года назад
GCM06: Throwing a ball vertically under quadratic drag
GCM05: Projectile motion with air resistance
Просмотров 9 тыс.2 года назад
GCM05: Projectile motion with air resistance
GCM04: Gauss' divergence theorem and Gauss' law for gravity
Просмотров 4542 года назад
GCM04: Gauss' divergence theorem and Gauss' law for gravity
GCM03: Conservative forces, potential functions, and Stokes' theorem failure!
Просмотров 2922 года назад
GCM03: Conservative forces, potential functions, and Stokes' theorem failure!
GCM02: Vector algebra and vector calculus
Просмотров 3182 года назад
GCM02: Vector algebra and vector calculus
GCM01: Linear and angular impulse of a Yo-Yo
Просмотров 8732 года назад
GCM01: Linear and angular impulse of a Yo-Yo
UP1 (10B-4) Moments of inertia in 1,2&3 dimensions with a single linear density
Просмотров 1772 года назад
UP1 (10B-4) Moments of inertia in 1,2&3 dimensions with a single linear density