
- Видео 36
- Просмотров 52 934
Manifolds in Maryland
США
Добавлен 12 фев 2021
Exploring the fascinating world of geometry.
Kickstarted by Tamás Darvas (@tamdarv), the channel features insightful contributions from Jakob Hultgren, Dan Horstman (@howtuts6591), Elliot Kienzle (@chessapigbay), Yanir Rubinstein and other members of the Department of Mathematics at the University of Maryland.
Partially supported by the NSF.
Kickstarted by Tamás Darvas (@tamdarv), the channel features insightful contributions from Jakob Hultgren, Dan Horstman (@howtuts6591), Elliot Kienzle (@chessapigbay), Yanir Rubinstein and other members of the Department of Mathematics at the University of Maryland.
Partially supported by the NSF.
Scott Wolpert on not hearing the shape of the drum
For full interview see ruclips.net/video/r5eJC0QFVgc/видео.html
Просмотров: 180
Видео
Scott Wolpert's advice to young geometers
Просмотров 2705 месяцев назад
For the full interview see: ruclips.net/video/r5eJC0QFVgc/видео.html
Beneath the Surface - interview with Scott Wolpert
Просмотров 3005 месяцев назад
In the first episode of Beneath the surface, we interview Scott Wolpert. Yanir Rubinstein chats with Scott about math, magic, music, and Riemann surfaces, hearing (or not hearing) the shape of the drum and hyperbolic geometry. Scott also talks about his hobbies. Prof. Wolpert's professional website: www.math.umd.edu/~swolpert/ Prof. Wolpert's RUclips channel: www.youtube.com/@UC5_OKn5fpweanMsQZ...
Visualizing Conic Sections Using Blender and Desmos
Просмотров 502Год назад
In this video we take a look at the ellipse, parabola, and hyperbola through the perspective of conic sections. I utilize the free open source 3D modeling and animation program Blender and the free graphing calculator Desmos. My beginner Blender tutorials: www.youtube.com/@howtuts6591/featured Blender: www.blender.org/ Desmos: www.desmos.com/calculator
Rotations in 3D Graphics With Quaternions
Просмотров 13 тыс.Год назад
In this video we will explore the advantages of using quaternions to calculate rotations in three dimensions. For examples we utilize the open source 3D modeling and animation software Blender as it has has several options for doing rotations. It can be downloaded free here: www.blender.org/ I do beginner Blender tutorials on my other RUclips channel here: www.youtube.com/@howtuts6591/playlists...
Geometry With Compass and Straightedge
Просмотров 280Год назад
In this video we will do some geometry like the ancient Greeks using just a compass and straightedge. We cover the basic rules of using these tools and go over several types of constructions. Chapters: 0:00 Introduction 0:37 Tools Required 2:03 Rules of Compass and Straightedge 3:36 Equilateral Triangle 5:25 Bisecting a Line 6:06 Drawing a Parallel Line 6:45 Inscribing a Hexagon in a Circle 8:5...
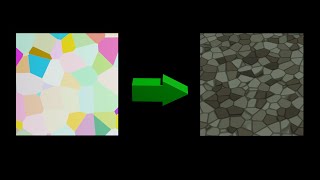
Using Voronoi Diagrams In Computer Graphics
Просмотров 303Год назад
In this video we explore Voronoi Diagrams and their applications in computer graphics for creating textures and randomizing geometry. This video makes use of Blender, a free open source 3D modeling and animation program: www.blender.org/ I also make beginner tutorials for Blender here www.youtube.com/@howtuts6591/featured
Normal Vectors and Their Applications in Computer Graphics
Просмотров 2,8 тыс.Год назад
This video discusses normal vectors, how they work, how to calculate them, and a variety of uses in computer graphics including baking normals on a plane to fool the lighting system into thinking there is more complicated geometry on the 3D model. For the demonstrations we utilize Blender, a free open source 3D modeling and animation program: www.blender.org/ I also have another RUclips channel...
Euler's formula and spherical geometry
Просмотров 374Год назад
We present Euler's formula for convex polyhedrons and give a proof using spherical geometry.
The Bergman kernel of the polydisk and the ball
Просмотров 267Год назад
I compute the Bergman kernel of the unit polydisk and the unit Euclidean ball. For my previous video on the Bergman kernel see ruclips.net/video/loIC28LNgNM/видео.html
What is the Bergman kernel?
Просмотров 520Год назад
I introduce the Bergman kernel of a domain and study its first properties. For more on this topic see Chapter 1.4 of Krantz's "Function theory of several complex variables."
The Cartan-Hadamard theorem
Просмотров 9092 года назад
I give a proof of the Cartan-Hadamard theorem on non-positively curved complete Riemannian manifolds. For more details see Chapter 7 of do Carmo's "Riemannian geomety". If you find any typos or mistakes, please point them out in the comments.
The Hopf-Rinow Theorem
Просмотров 9572 года назад
We present a proof of the Hopf-Rinow theorem. For more details see do Carmo's "Riemannian geometry" Chapter 7.
Lie derivatives of differential forms
Просмотров 6 тыс.2 года назад
Introduces the lie derivative, and its action on differential forms. This is applied to symplectic geometry, with proof that the lie derivative of the symplectic form along a Hamiltonian vector field is zero. This is really an application of the wonderfully named "Cartan's magic formula" The invariance of the symplectic form almost immediately implies Liouville's theorem, an important result in...
Poincare recurrence
Просмотров 6922 года назад
I talk about Poincare recurrence, inspired by Louiville's theorem in classical mechanics. Previous videos in this series: Lie derivatives: ruclips.net/video/8hWOHJ4Xka8/видео.html Louiville's theorem: ruclips.net/video/YABAJS3FIEA/видео.html








Can you please make a lecture on Levi flat part of boundary of a domain in C^n
Sehr gut
the graphics are amazing. how did you make them?
Interesting presentation. Well done.
a vector can be a single number if you live in 1D
I think considering reflexivity in the equivalence relation of cobordant manifolds gives some justification for why in A^1-homotopy theory the affine line A^1 replaces the interval [0,1]. A cobordism theory with this equivalence relation using the affine line describes an algebraic cobordism. The algebraic cobordisms between complex manifolds are of most interest since polynomials are holomorphic (= infinitely complex differential). Note that solutions to sets of polynomials are algebraic varieties.
thanks for these valuable remarks!
@@manifoldsinmaryland Yup 👍. I think I found a better reference for why the affine line A^1 is used in the notes of “Motives I” by Eion Mackall. There near the end he mentions: “…the cohomology we work with satisfies the property H*(X x A^1) = H*(X). One way to see this is the calculation H^1(A^1) = 0 and then use the Künneth decomposition on the product. …we consider A^1 as a suitable substitute for the interval’s role in homotopy theory.” Thom’s theorem equates cobordism to a homotopy theory. Thom’s theorem derived that cobordisms groups are homotopy groups of (universal) Thom spectra MG by π_n(MG ⋀ X) = MG_n(X) with G a topological group such as the unitary group U. The associated cohomology theory of a spectra E is of interest considering the realization functor mentioned in 4 of Mackall. Whitehead showed that any homology is E -> π_(E ∧ X) = H_n(X; C(X)) for spectrum E on CW-complex X with coefficients C(X). Brown’s representation theorem or assuming Poincaré duality between homology and cohomology (ie Weil cohomology realized by motive of rational equivalence) it is possible to derive a cohomology theory from a spectrum E such as the Thom spectrum MG.
Splendid !
at 23:30, the star of the poincare duality and the use of Hodge star operator are used in the same place, kind of confusing.
true, I wish there was something I could do about it :)
@@manifoldsinmaryland Anyway, great structral explaination and thank you for the video! I also did a talk on the Hodge Theory on Monday :)
Glad you found our summary useful.
Omg thank you so so much 😭😭💖💖💖💖there's a vfx artist who said it would be a plus learn this topic, so here am I, and you explained it perfectly 😭💖💖💖💖
thank u for this awsome video I have been trying to cauculate the normal Vectors for my 3d terrain generator on the GPU and all the methods didnt work exept your method. Great Video!
Amazing video ,not just directely giving definition
complex analysis is dope
Cool!
Nice one brotha ☀️
Cool. I just try to recall my study subject. Thanks for sharing.
Useful info, thanks!
7:24 why is a closed segment cobordant to zero? would the cobordism not have to meet its endpoints with edges and thus create more boundary than just the segment, making it not a cobordism?
nevermind, it's because a closed segment isn't a manifold. which is stupid, but true nonetheless lol
True. Thanks for visiting the cobordism exhibition!
so intutive
Phenomenal!
Hodge is long gone, and turning over in his grave because of the hodge-podge this old fart has created out of his ideas.
What background do I need to understand this? Learning differenential forms now. I know very basic stuff about manifolds e.g. tangent space/bundle. I don't know differential geometry.
That should be enough to get started. A good book that is suitable for your background is Wells' Differential Analysis on complex manifolds
@@manifoldsinmaryland I didn't know if you would answer so I didn't give that much info. Let me give a little more. I want to understand the generalization of Helmholtz decomposition to n dimensions. Wikipedia says that's called Hodge theory. I don't think I need too much about *complex* manifolds specifically but maybe that helps. I'm from economics / game theory not physics/math. Does your book recommendation still stand? OR is there a better book in that case? Thanks!
@@alexboche1349 It does not stand anymore. Unfortuantely, I know little to nothing about Hemholtz equations :(
Yo who else is watching this video because of our CALC 3 class?
Desmos 3D got released a few days ago!
thank's very usefull and informative insight ! is there any reason good not to use quaternions then ?
too hard to learn ;p
one reason is that geometric algebra is considered even better way to rotate (but also somewhat harder to grasp), so why no learn it instead haha
Wow great video, it's just that it was too advanced for me to understand all those mathematics for my simple brain. Can we get a video with simpler explanations with examples to visualise what's being said?
Hey there, that an interesting explanation, I would love to see more of utilizing blender and general physics to understand complex topics visually❤❤❤❤❤❤
nice video!
Crystal clear explanation, thanks doc.
Beautiful aesthetic to this video! I especially loved seeing the tile floor!
Wow, this is legit amazing work. More people need to see this, it is very well made 👏👏
Thank you so much 😀
Really cool stuff. I wonder if this could help with projections of surfaces (e.g. a tube or torus) onto a 2D plane - help to distinguish between the points which are closer and points which are further from the viewer?
Nice proof
ey good video, a have a quastion, which is that sofware??
Good video, subscribed
Wow! Excellent! Thank you.
Glad you liked it!
These are wonderful videos. Thank you so much for them!
You're very welcome! Thanks for watching.
Extremely helpful!
Glad to hear!
Nice video very clear explanation, can you make a video on riemann metric and curvature on manifold
sounds like a good idea.
I am looking for that thanks keep it up.
Thank you!
At 3:08 what is $X$? Is it $\{(z_1,\dots, z_n): (z_1,\dots, z_{n-1},0)\in L\cap \Omega, z_n\in \mathbb{C}\}$? We probably need to be more careful about the cutoff then.
Thanks for noticing. X should be simply Omega here. Indeed, I should have said more about the construction of rho, but I am never really sure how much details is OK in math RUclips videos.
@@manifoldsinmaryland How can $X$ be $\Omega$ if the section $L\cap \Omega$ is small ? e.g. take $\Omega$ to be a ball in $\mathbb{C}^2$ and let $L=\{z_n =0\}$ be a complex line that does not pass through the center of $\Omega$ but intersects $\Omega$ in a nonempty open set. Then the formula $g(z_1,\dots, z_n)= f(z_1,\dots,z_{n-1})$ does not define $g$ on all of $\Omega$, but on a proper subset.
@@debraj10 not sure what you mean. I say specifically that g is defined on a proper open subset of Omega. Then I construct the global extension using a cutoff of L cap Omega.
It should be (Graph of - T)^perp around 7:40
Where we are using exp_p is defined for all of T_pM when we are proving there exists a distance minimizing geodesics
For v, the limit of the v_i’s
indeed!
This is wonderful
Hi how are you?. I would like to know, what difference can exist between a compact Kahller manifold in X, with the HyperKahlerian manifolds, one of the cases that I consider is that for HyperKahlerian metrics X can be compact in a $\textbf{P}^{5}$-hyperplane special of the Theorem of Lefschetz-Noether, , But in an article of mine (if you want I'll pass it on to you), I was able to prove that in HyperKahlerian metrics, X is not only "compact" but also linear in $X\to{} g_{ij, k}$ where for example $X_{1}\xrightarrow{\ \sim\ }g(1) (See X1 as a Linear-Isomorphism on a generic-Kahller Einstein in g(1)) So a compact X becomes in $X+ g_{ij, k} Where, this linear-isomorphism of g(1) is a HyperKahlerian metric, which is in d= g_{ij, k}> g_{i, k} -g_{j} where it differs, only by a semi-stability, to the Kahller-Hermitic metric, In this case the compact of X is a special hyperplane, which depends on any d-degree with singularity on a curve C(g(1)) (see you also as if $X\subset{} \{\mathcal{M}_{g, d}= g_{ij, k}$) Where, well, the Noether-Lefschertz theorem of $\textbf{P}^{5}$ is generalized, for any $d\geq{3}$, with D-degree in the singularity of a curve, for $d>3+ d<3,$ in this case is true for example that S5, S8, S(7,8) and Sg (6,9), can produce forms of that hyperplane of NL, and are identical to a HyperKahlerian metric on X, In this case we can write to X(g(5, (7,8))$ where X_{1} as the structure compact of an isomorphism in the Metric Hermitic-Variety Kahller for example in d<0= k[I, J]- (I, J) (see the square bracket of a quaternion, for Lie-algebras), The idea is in $X_{1}$ is true an theorem of Brill-Noether? if only considered an idea for fixed-curve invariant-Donaldson and Thomas?, then, is a DT-invariant for an irreducible B-model also a hyper-Kahllerian metric, too? and I got this question too, and if it is true because a DT-invariant is equal to 0, in A-reducible models?. This is part of a general model of how these very high-dimensional manifolds can generally be differentiated.
ooh found this via the recommender
check back for more!
this is really cool, thanks. Any chance you would cover Chern-Moser theorem on normal forms of hypersurfaces?
thanks for the suggestion!
@@manifoldsinmarylandI agree with Czeckie.a vid of Chern Moser normal forms would be fantastic!
Hi Firstly thanks for such amazing videos Can you please make a video on Kahler identities? There's this operator called lambda which is adjoint of the operator L ( L is the operator that wedges with the Kahler form). Can you make videos on that please?
thanks for the suggestion! I will put that on my list
Very Nice Summary. Please make more video to explain maths
We will!
What an incredible video! I was reading about cobordisms in the context of TQFT and found this gem. I wish I could visit the Cobordism Exhibition in real life...
Glad you enjoyed it!
Good can you make a talk, about the Hodge conjecture, I am working the CH for example for a dimension p-addic "convergences-thus falls" where the CH is true only under a 2-cycle Hodge, because I found that p- adic ($P= N_{|-1|}$ as an isomorfism of the algebraic in $N\subset{} K$) where the $Map_{K}=1$ an also this contains to $Map_{K}: \sum_{j}^{\infty= - i}$ where the P-adic case on the dimensión $q(2n)> p$ it is well a complement with the subvariety (n-1) where the contains "symmetric" is localy finite in $\delta\mathfrak{H} \to (\delta, - \delta)$ (which takes for a finite thing, its value in the regular Homotopia), Where if the Homotopy coincides with Mod p (1), then a discrete set of normal walls is produce.
Thanks for the comment. Unfortunately I know very little about algebraic geometry over the p-adics.
@@manifoldsinmaryland Yes, of course, but knowing things about what a 2-cycle is, for example from Hodge, your P-adic space can be an application of how this is continuously pasted into a complex N_ {| -1 |} submanifold.
@@manifoldsinmaryland For example, you can consider the space P-adic (which in general is $Q^{*}(H)$-adic or also as $Q^{*+n}$-adic of cycle ) On some standard volume (group SU3 in the hyperplane convex) , which corresponds to a Hodge-structure, I for example have been studying the P-adic for Hodge- 2-dimensional structures, like $ P: Hdg_ {2} here you see that also P \ xrightarrow {\ \ cong \} Hdg_ {2}, where well any P-adic volume of a Hodge-structure is identical to an isomorphism -linear, Thanks to this dear colleague, it has been possible to generalize the P-adic volume (see it as a Module-potent oin SU_{Z3} =Mod-p^{2} (x;i)) where all the Q(H,n)-cycles of structure linear are compatible with the linear part of some P-adic volume, from here for example the Hodge-conjecture arises but in this case for example the P-adic is wrapped in P = | -1 |\ in {} N (see N as the submanifolds of N_{g^{p,q}}$) where the P-adic corresponds to a Hodge structure of class q (2n)> p (case of 2 Hodge cycles), where well the volume of all Hodge structures is generated in a semi-stable abelian manifold, see how P (adc) = N_ {| -1 |} \ to \ log_ {q} (where \ log_ {q} = \ log_ {, (2n) - xi}) which is where all logarithmic abelian manifolds admit some volume "in q" of the structures of 2 -Hodge cycle, for example, you can write to q: = P * = P (adic). Currently, I am working on a paiper, (which if you want I can send you), with the CH (conjecture-Hodge), for P-adic which are associated functions of P_ {l <0} (where l is some cohomology group in Hdg_ {2n}), my approach is that for the spaces $ P_ {l <0} - $ adic the preserved volumes (see it as an indicator of symmetry) are true for a subgroup of functions l g3 = 1, or if the P_ {l <0} \ to g3 = Mod 1 where well all the projections of a subgroup in 3 are relevant, in the volume P_ {l <0}, this case for example leads us to think of the previous idea as the "extension "of a semi-stable manifold $ \ log_ {q} $ must be identical to $ q (2n)> p $ geometrically representing the P-adic in this case, only as a volume of symmetries locally. This as the volume of symmetries locally is this maximum smooth subgroup g3 in a local finite Map_ {K} = (n-1). That is why I tell you that the P-adic space of a dense volume of symmetries is of intense understanding within the CH, and Hodge's own theory. So my general version of the, CH is the following consider whether $g3 \{ Mod |p, +1|\forall{} N_{|-1|}\} or g3= Mod|p, +1|\times{} \mathcal{N}_{|-1|} where see you that, the $\mathcal{N}_{|-1|}$ of an structure of submanifolds in $P_{*}$ (see you only as $\mathcal{N}\subset{} P_{*}$) is the standard case of, admitting P-adic in some N submanifold, Even since the P-addiction is of a soft or projective class, aEven since the P-addiction is of a soft or projective class, is traced a $P(adic)= P_{('(2n)+1)} but if $P_{*}\in{} N_{|-1|} then you written to $P(adic)= P_{(, (2n)-1)}$ or also written as $P(adic)= P_{l<0}$ where l are all dense and maximal correspondence functions, identical with modulo in a submanifold n-1, Proving that the CH is only true if g3 = Mod | p, +1 |. but not in 3 nor its present dimensions. I await your answers, if you want me to send you my paiper. greetings friend
100% a crank