
- Видео 41
- Просмотров 74 470
Let's Get Complex
Германия
Добавлен 7 сен 2021
Just a guy who is trying to learn and share
De Moivre's Formula | How to Find the nth Roots of A Complex Number
In this video I show you how you can change a complex number from the rectangular form to the polar form using the Euler's formula. Then I introduce the De Moivre's formula which helps us to easily take a complex number to powers of n and finally we try to use this formula to find the roots of a complex number.
00:00 Complex Numbers: Rectangular Form Vs. Polar Form
03:34 De Moivre's Formula
05:43 Nth Roots of A Complex Number
00:00 Complex Numbers: Rectangular Form Vs. Polar Form
03:34 De Moivre's Formula
05:43 Nth Roots of A Complex Number
Просмотров: 545
Видео
Complex Multiplication in Terms of Dot Product and Cross Product (with Geometrical Interpretation)
Просмотров 330День назад
In this video we first talk about the definitions and geometrical interpretations of dot product and cross product and then see how they are related to complex numbers. As we will see the multiplication of the conjugate complex of a complex number with another complex number yields a new complex number whose real part is the dot product of the associated vectors and also the imaginary part is t...
Can Math Be Beautiful? The Mystery of Euler’s Identity
Просмотров 2,2 тыс.21 день назад
In this video, we delve into the beauty of Euler's Identity, often celebrated as the most beautiful equation in mathematics. We start by deriving Euler's Formula through the Taylor Series expansion of exp(i*pi), graphing the first 10 terms to reveal how it reaches minus one. This journey leads us to Euler's Identity: exp(i*pi) 1 = 0 We also explore the modulus and complex conjugate of complex n...
Are Complex Numbers Just 2D Vectors? Here’s Why Not! (with Visual Examples)
Просмотров 11 тыс.21 день назад
I suggest you to watch this video to get a better understanding of the relationship between the complex multiplication and the dot and cross product: ruclips.net/video/UuY4n22ZEl4/видео.html In this video, I explore the fascinating differences between complex numbers and vectors. I’ll start by graphing some complex numbers and adding them on the 2D plane, showing how they can look and behave si...
Complex Numbers | Basic Operations (Addition, Subtraction, Multiplication, and Division)
Просмотров 56428 дней назад
In this video we add, subtract, multiply, and divide complex numbers to see different basic operations with them. Addition and subtraction are quite the same and for multiplication and division we need to know some important properties that you can see in the examples. For example, i squared is minus one which is a real number. The important thing about complex numbers is that we can write them...
Math teachers betrayed us all! | An introduction to complex numbers
Просмотров 538Месяц назад
In these series of videos, I'm going to talk about complex numbers and show you how we can use these amazing numbers. When you solve a quadratic equation using the famous quadratic formula, if the discriminant is negative, we have two complex numbers which are amazing. They have a real part and also an imaginary part. The real part is familiar, but the imaginary part is a real number multiplied...
Dirac Notation (Bra-Ket) | Understanding the Maths of Quantum Mechanics
Просмотров 533Месяц назад
In this video I start by making an analogy about our emotions as emotional states and continue to introduce a powerful and compact mathematical framework that makes it easier for physicists to work with quantum mechanics. This notation was introduced by Dirac and is also known as Bra-Ket notation. I start by introducing ket and bra vectors which are elements of the Hilbert space and then operat...
Linear Motion: Distance, Displacement, Velocity, Speed
Просмотров 732 месяца назад
In this video I introduce some basic concepts in physics like distance, displacement, velocity and speed. We introduce average velocity and average speed and graph a moving car using an x-t diagram.
Solving the Schrodinger Equation in 3D | Infinite Spherical Well (Spherical Bessel Functions)
Просмотров 4102 месяца назад
In the previous video, we talked about the angular equation derived from the Schrodinger equation in three dimensions (3D). In this video, I talk about the radial part and as an example, I solve the radial part for the infinite spherical well for two cases: when l is zero and when l is not zero. We also talk about the effective potential and see how useful it is in solving the Schrodinger equat...
Solving the Schrodinger Equation in 3D | Angular Equation (Spherical Harmonics)
Просмотров 1,5 тыс.2 месяца назад
In this video I have talked about how we can solve the angular equation part of the Schrödinger equation. The solutions are spherical harmonics and based on the l (total angular momentum or azimuthal quantum number) and m (relating to z component of angular momentum called magnetic quantum number). This is the first step, actually the angular step towards finding the solutions of the Schrodinge...
Scalars and Vectors (with 4 practical examples in physics)
Просмотров 2332 месяца назад
In this video, I have introduced scalars and vectors and used four examples to show you how we can use them to quantify our physical world. Scalars are just represented by a magnitude, while to show vectors, we need a magnitude and also a direction. In the 4 examples I have shown you how we use these quantities in Newton's second law, universal law of gravitation, kinetic energy and the Hooke's...
Don't Study Physics, Start Wondering!
Просмотров 5343 месяца назад
In this video we explore the concept of having a standard unit for speed and how we can change other units or scales to meters per second which is the accepted SI unit. The beauty of science is that people try to find common grounds so they can understand each other better, and in the heart of this video you can see this endeavor.
Dimensional Analysis | This is how we give meaning to numbers
Просмотров 4473 месяца назад
In this video I talk about 7 base units we use when we want to measure physical quantities. These 7 base units or dimensions are meters (length), seconds (time), kilograms (mass), amperes (electric charge), mol (amount of substance), kelvin (temperature), and candela (luminous intensity). All the other units in physics can be written using these 7 ones. Pay attention to check the dimension of b...
A beautiful solution using binomial expansion and the product rule
Просмотров 4603 месяца назад
In this video I show you how you can differentiate higher order derivatives of multiplication of two functions. First, we begin with the binomial expansion and also Pascal's triangle and then move on to the product rule and combine these methods to find a solution for this derivative. Finally, I talk about a possible computational functionality of by defining a function which seems interesting ...
A Brief History of Numbers
Просмотров 6386 месяцев назад
In this video I have talked about Numeral Systems. How did people use numbers in the past? How have we come up with using this kind of decimal system we use today? I start from about tens of thousands years ago when they used some bones (Ishango bone and Lebombo bone) as mathematical tools to keep track of the days in a month. Then, I start talking about Sumerians and Babylonians who were the f...
Solving the Schrodinger Equation in Three Dimensions | Deriving Angular and Radial Equations
Просмотров 1,5 тыс.Год назад
Solving the Schrodinger Equation in Three Dimensions | Deriving Angular and Radial Equations
Deriving Spherical Coordinate Unit Vectors (with Geometric Interpretation)
Просмотров 18 тыс.Год назад
Deriving Spherical Coordinate Unit Vectors (with Geometric Interpretation)
Order Matters? (Commutation Relations in Quantum Mechanics)
Просмотров 509Год назад
Order Matters? (Commutation Relations in Quantum Mechanics)
The Schrodinger Equation in Three Dimensions
Просмотров 1,7 тыс.Год назад
The Schrodinger Equation in Three Dimensions
All You Need to Know About Vector Operations (Including Their Geometrical Interpretation)
Просмотров 160Год назад
All You Need to Know About Vector Operations (Including Their Geometrical Interpretation)
Position Space and Momentum Space Wave Functions
Просмотров 1,3 тыс.Год назад
Position Space and Momentum Space Wave Functions
The brain is cool, not a cooler Aristotle!
Просмотров 252Год назад
The brain is cool, not a cooler Aristotle!
Hilbert Space | Mathematics of Quantum Mechanics
Просмотров 7 тыс.Год назад
Hilbert Space | Mathematics of Quantum Mechanics
Solving the Schrodinger Equation | The Free Particle
Просмотров 1,9 тыс.Год назад
Solving the Schrodinger Equation | The Free Particle
Linear Algebra | Linear Transformations and Matrix Representations
Просмотров 143Год назад
Linear Algebra | Linear Transformations and Matrix Representations




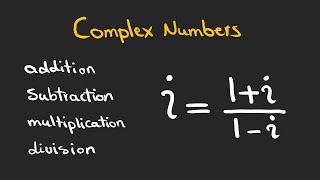




i like the begining where u put emotion, it makes it easier to understand.
I'm glad that you found the analogy useful.
Let's decompose the euler equation,, e^(iu) + 1 = 0 ,, note u = pi || 2e^(iu) - e^(iu) = e^(iu) || 2 - 1 = 1, so || 2e^(iu) - e^(iu) + 2 - 1 = 0 || 2e^(iu) + 2 - e^(iu) - 1 = 0 || 2(e^(iu) + 1) - 1(e^(iu) + 1) || we have two (e^(iu) + 1)'s, so we use only one giving us || e^(iu) + 1 = 0 and 2 - 1 = 0 || e^(iu) = -1 and 2 = 1 ||
In the last part you have (2-1)(e^iu+1)=0. So either the first or the second parenthesis is zero. Obviously (2-1) is not zero, so (e^iu+1) must be zero which is the euler's identity.
@getcomplex That's ✅
💛
@@getcomplex 2(e^(iu) + 1) - 1(e^(iu) + 1) = 0 || e^(iu) + 1 = 0 ~> e^(iu) = - 1,, -1 + 1 = 0 || 2 - 1 = 0 ~> 2 = 1,, 2 - 2 = 0 ,, 1 - 1 = 0 || (2 - 1)(e^iu + 1)=0 ~> 2e^iu - e^iu + 2 - 1 = 0 ~> e^iu + 1 = 0 || u = pi
Excellent video. Thank you!
Thanks. Glad you liked it 💛
elegance.
💛💛💛
bro pls post more iam a cs student and i need this if you have a discord or any way to contact you please let me know
Happy to hear it's been useful. Send me an email. You can find it in the descriptions of the channel.
Awesome😊
Thank you. Happy you liked it 😍😉
Shame about the annoying, unnecessary background noise that distracts from the important narrative. For this reason I am unable to share this instructive video.
I'll drop the background music in my upcoming videos. I agree that it might be annoying. I was just experimenting and although the figures have gone up but I find the background music distracting myself, too. Thanks for sharing your opinion.
@@getcomplex Many thanks - I have just become a subscriber.
Happy to hear that. I hope you watch and enjoy the upcoming videos 💛
very well explained. good job
Thanks bro 💛
A great video
Glad you enjoyed it
Did bro just summarize my 3 years of quantum mechanics courses in 4 min? Awesome!
Bro has just started making videos and is happy you are watching them 😄💛
Hey was wondering if you could make a video on differential equations I loved ur style of teaching
Maybe even a series of videos as a playlist. Nice suggestion. I'll do it.
loved your presentation
Glad you liked it 💛
What i loved most about this video is the fact that you’ve not only derived the formula but made a deep connection with the history and the meaning as well. To me that’s almost as beautiful as the formula😀
Wow that's such a kind compliment 😍 I'm happy you liked it Arda.
Its complex time
It actually is 💛
How do you make your videos.
Hey Nazish. I use powerpoint and its animations. With a voiceover on the slides being screen recorded.
Beautiful presentation
Thanks a lot. Happy you liked it
Thanks for this
You are welcome. 😍
this identity is truly beautiful which connects real and imaginary numbers ❤
It really is!
Thanks! Great summary and helps while reading quantum mechanics texts!
Glad it was helpful!
Hi friends, I hope you are all fine. My explanations in this video seems to have caused some confusion. I'm going to make another video (ruclips.net/video/UuY4n22ZEl4/видео.html) on this playlist (and then pin it in the comment section and description) to fully explain what the problem is. I'll include some of the comments on the video and will talk about them. I suggest you to read the comment section if you have watched the video. There are lots of good comments that I myself learned a lot from. Feel free to leave new comments and share your thoughts. It's emotionally really difficult to me to deal with some comments especially when I know I had a part in this confusion, but I feel responsible for all the people who watch this channel and try to learn something. I emphasize that I'm just a guy who is learning and sharing some stuff I'm passionate about and I can assure you that you are going to see more quality content from this channel in near future and will learn a lot of things either through my videos or even better through the mistakes I make in some of them.😀 Love Let's get complex...
Hey I just wanted to let you know that I enjoyed your video. At the end of the day it's all semantics and pedantic. People looooove being technically correct especially on the internet. Don't let yourself being dragged down. I know where you were comming from. You can look at it from different perspectives. If you look at certain aspects like scalar multiplication and Complex Number Multiplication of course that is different. if you want to definie a multiplication in R² like (a,b) * (c,d) = ( ac - bd, ad + bc ) then you can show it's isomorphic to the complex multiplication. But I agree. The way Vector Spaces and Complex Numbers are introduced in school they are different beasts. Keep up the good work ^-^
@SimchenThank you so much for your heartwarming comment. I really appreciate it. I'm working on this channel beside my work which is not even related to physics or math. My job takes me about 50-60 hours a week and this channel is where I talk about my passion. Despite all the different comments I got on this video, I should admit that I learned a lot and what I am truly trying to show here is my effort towards what I love and this is the journey I have just begun amd will continue for the rest of my life. In fact, a kind of documentation of my journey. Again, thanks for your comment. Really means a lot to me.
Please people stop watchin this kind of math education, it only brings up confusion. The author obviously does not know how a vectorspace is definedi nor does he know what is an euclidian vektorspace, nor does he know what a field is, and he is playing around with multplication symbols, thats all, people buy a book and learn it the hard way by READING.
I wholeheartedly accept your criticism and admit that my explanations might have caused some confusion, especially in the part I was talking about multiplications. As a physics graduate, I have some knowledge about the topics you mentioned, but I couldn't fully explain what I meant which show I should be more careful about making my next videos. In this video I was trying to show that complex numbers are not JUST like vectors and I clearly didn't talk about other forms of multiplication. I'm going to create another video and include some good comments under this video (including yours for the beginning of the video to roast myself 😄) and explain more about what I had misleadingly presented. And I totally agree with you on buying books and reading from the source itself. I have tried to stick to these sources so that I don't cause any confusion, but sometimes I miss things. Some of the comments under this video have been a wake-up call to me in order to make better videos in the future. Sooo, the bottom line is that I take full responsibility for my videos and try to make better videos which are clear and thorough in the future. Thanks for your time
Why do you claim that multiplying vectors is the same as their dot product?
By that part of the video I meant that we don't have the algebraic multiplication for vectors. But it seems that my explanation is a bit misleading. In this video I was trying to justify that complex numbers are not just vectors, but more.
@@getcomplex should check out geometric algebra... has a way of multiplying vectors which doesn't reduce down to just the dot product but that is a component of it. And it unifies complex and vector algebras into one algebra. One product to multiply vectors or imaginary numbers or even things like quaternions
Thanks for the suggestion. Yeah I checked and learned geometric algebra and it is really nice. I have made another video talking about multiplication of complex numbers which you can find in the caption of the video and in that I have made it more clear and also shown how we can find the area of a polygon using complex multiplication. I really appreciate your time for letting me know about these useful information you have. I'll definitely study quaternions later.
pedantic and stupid. everyone knows every field is a vector space over itself and C is a division algebra (i.e. a kind of vector space).
Yeah you are right, but seem to be very angry. It's not worth it my friend😄. It's just a bad video as you say and by reading your comment I might have learned something new, so thanks for your information, but not your tone.
His explanation is very interesting
Glad you liked it.
tan (2/3 radians) != tan (33 degrees). 2/3 radians are 38.197 degrees
No, but the two spaces are isomorphic.
...under addition.
Vector represents level of students life who takes maths or physics or engineering in higher studies Level 1 noob: vector has magnitude and direction Level 10 amateur: vector is a component of vector space Level 99 boss: vector is a rank 1 tensor :')
Level million plus, there are no vectors any more, just branes😂😅😮
🤓🤓🤓
😄👍
Vectors is a legit math tool since it deals with countable real numbers. On the other hand the imaginary numbers aren't legit since they aren't countable and violate basic math rules. I know what modern academia says but it's all bs.
Yeah I see. I actually don't know what modern academia says, but I'm trying to learn some science stuff and make videos about them. I've learned a lot from some nice people commenting under my videos and I find this process an amazing learning experience. And it is helping me to even make better videos and improve.
The complex number form a vector space over the real numbers, or over the complex numbers. They have lots of other properties, but they have all the properties required to be a vector space. In general any field can be a vector space over itself.
Thanks for your explanation.
such a wonderful explanation....man...amazing
Glad it was helpful!
great video man....pl post more...
Thanks, will do!
I find populism in mathematics disgusting. What does the number "i" model in mathematics? Answer:👉 models rotation. You rotate clockwise with "i" and counterclockwise with "i". This does not prove that the number "i" is real. proves that rotation is a real event. The fluidity of forgetting the model nature of mathematics and substituting the model for reality is a modern form of bigotry. We can also use the following alternative model for a 90 degree counterclockwise rotation, that is, for multiplication with "i". We can define the following for (xy) pairs in the coordinate system: (a,b) = (-b,a), (-b,a) = (-a,-b), (- a,-b)= (b,-a), (b,-a) = (a,b), (4,1) = (-1,4), (-1,4) = (-4,-1) ,(-4,-1) = (1,-4), (1,-4) = (4,1) oops, looks like we broke your "i" idol. Because we are aware of the modeling nature of mathematics.😀😉😆
So why do these complex numbers work in quantum physics? Firstly, there is rotation in quantum physics: spin. ,the second is the wave function There are places in the square where the function is zero, and this is similar to waves canceling out each other. If a^2+ab+ba+b^2 has axb=- bxa, that is, if you do vector multiplication, a^2+b^2 You get it. Geometric algebra can do this with bivectors. This is a model. Now we will do the same thing with another model. (1+1i)(1-1i)= 1.1+-1i+1i-(1i.1i)=1.1+0i-(-1)=1+0+1 😉 These are all models, not reality, they are tools to model reality.. If it doesn't work for you, you can find a different model and throw away the old one.
Now why should we rotate with "i" when we already have a model that allows rotation in 2 dimensions? Now imagine that you multiply the (a+b) vector with the (a-b) vector in this model. (a+b) (a-b)=(a.a)-(b.b)= a^2-b^2. If we do this on the complex plane (a+bi) (a-bi)=a^2+b^2 We get it. Moreover, this exactly mimics the anticommutative property ordered by quantum physics. We ensure the order by using complex conjugation in 2 dimensions and doing a juggling trick. (a+bi)(a- bi) =a^2- bi+ bi- (bi)^2 I choose a=1, b=1: 1-i+i-(i)^2=1+0i+1. We have provided the quantum order, but the problem is this: (a^2-b^2) and a^2+b^2 are not the same. This is not the same as Hamilton's model, which rotates a,b pairs in 2 dimensions. For this reason, rotation with "i" There must be a rotation operation in 3 dimensions. More precisely, the process of reflecting vectors on two planes positioned at 90 degree angles is a rotation action. Like the electric and magnetic fields... we think of the electric field as horizontal and the magnetic field as vertical and at the same time turn clockwise with "-i". You will rotate it counterclockwise with "i".😉😎👍 We defined axb=- (bxa) in the vector product (you can also interpret this as rotating fields as bivectors, your interpretation is your model) You are modeling the same thing in the + bi- bi=0 operation. Remember the right hand rule, the thumb is the result vector and points to the 3rd dimension. (axb) = turning right, thumb up. (- bxa) turning left, thumb down. now (+ 1) thumb up,(-1) thumb down. (a+bi)(a- bi) =a^2- bi+bi- (bi)^2 ...do we see what we are doing with - bi and + bi? We do the labeling in the third dimension with +i and -i. What a big trick😀😅
Does this scalar value 3 in any way modify or influence the display of the 2D plane? or is it simply a tree that falls unheard in the woods?
Nicely mentioned 😄 As far as I know, the dot poduct is like a map that maps two vectors to a scalar which is a real number. The number 3 is that scalar.
I think what we can safely say is the dot product is not vector multiplication.
As far as I know it's a kind of vector multiplication. Maybe I should have also talked about cross product which is another kind of vector multiplication. To be clear, even cross product, which is another kind of vector multiplication is different from a complex number multiplication and the answer includes a combination of unit vectors that when looked at as one single vector is perpendicular to the planne in which our two first vectors exist. My main point in this video is to show that complex numbers form a field but vectors live in a vector space. And I can safely say that I tried my best to explain that and can also promise you that I'll try to get better at explaining things on this channel. Thanks for sharing your opinion and if you think I might have made a mistake in explaining the concept please tell me so that it is clearer to me.
I don't think we can safely say that without restricting what you're referring to as a vector. The dot product is a special case of the inner product (in this case taking two vectors to form a scalar), yielding an inner product space. All inner product spaces are vector spaces. The dot product is just a special (however common) case. To summarize, dot products don't exist without vector spaces (e.g. algebras) forming them. I am unsure why you're suggesting the idea of tossing out dot products as vector products to be a comfortable one.
I reccomend the videos on geometric algebra by mathnoma. He does a great job of talking about vector multiplication as the sum of the dot product and the wedge product (aka cross product). This flows seamlessly into complex numbers and into the law of sines and cosines. Really interesting videos and I wonder why this wasn't taught in my university math and physics degree. They are really excellent videos.
@@getcomplexAs others have pointed out, the biggest problem with this video is defining multiplication as the dot product. A much more natural definition of multiplication of vectors is the geometric product (the inner product+the exterior product). If you do this, and you have a Cl(1,1,0) Clifford algebra, then complex numbers become isomorphic to vectors and it makes more sense to say they are the “same”. (The 1,1,0 refers to the fact that one of your bases have to square to negative one while the other squares to positive one, which is also what makes complex numbers useful, but is not unique to them)
Yeah I got it. I just used the dot product as an example but it has made the video confusing. I'm making another video to show how complex numbers are related to dot and cross products so that it makes more sense. I don't know anything about Clifford algebra but I'll study it. Thank you so much for explaining.
Hello. Please answer me. How these videos are made, which applications do you use to make these math videos ? I see graphics, i see expressions, i see dynamism in the graphics etc . I just wanna know. Thanks
Hi I draw what I want to show on powerpoint and add some replay animations. Then record screen the presentation and speak on it. When you draw something in power point, it gives you an option in animations called replay which draws your strokes on the screen.
@@getcomplex So these dynamic math videos are made on PowerPoint ?
Yes. Just power point animations.
And sometimes I use photoshop and camtasia too. But mostly power point animations
@@getcomplex I wanna publish some new math concepts on RUclips. Is it a good idea or I should do a paper for a journal. I learned English recently for that. I'm francophone from Mali. Please answer me
Why can't we just define a vector space with the basis {1, i}, but the scalar field is the real numbers? To have a vector space we only need 8 axioms and none of them is about the inner product (there is nothing about any kind of vector-vector multiplication, there is only scalar-vector multiplication). Isn't the inner product just an additional structure and not mandatory for a vector space?
We can do what you said, but it would still be different from a field because of the multiplication. As you said the inner product is an additional structure and not mandatory for a vector space, it's not a built-in structure. However, in the structure of a field we have a built-in multiplication and that's where we can say complex numbers are different from vectors. It was the point I was trying to make.
A vector space with basis {1,i} and field ℝ is indeed ℂ, but that sells ℂ a bit short. What makes the complex numbers special and not just ℝ² is the multiplication. The complex numbers are equipped with a product which makes them an algebra. An algebra over a field F is an F-vector space equipped with a bilinear product. A bilinear product is a product that multiplies two vectors to get another vector (and follows some other rules). ℝ³ equipped with the cross product is an example of an algebra. ℝ² equipped with the bilinear product × such that (a,b)×(x,y) = (ax-by,ay+bx) is another algebra and is basically what the complex numbers are. But the complex numbers aren't just an algebra, either. They have an identity element under this multiplication, and all the nonzero complex numbers have inverses under this multiplication, and this multiplication is associative and commutative. All this together with the fact that (ℂ,+) is a group and that the product is distributive over addition means that (ℂ,+,×) is a commutative division ring, which is also called a field. So ℂ is a vector space, but it's also so much more.
Amazing explanation. Thank you so much. I'm going to dig deeper into these concepts like sets, groups, maps, rings and fields. And maybe share what I learned as a new post. Really appreviate your time wriring a thorough explanation 🙏
@@getcomplex In the video, you simply showed that if someone thought the product of vectors is the same as the dot product, they are mistaken."
@@thes7274473 Could you recommend a source to learn this ? This sounds super interesting but for someone like me totally unreachable with my current knowledge.
I know how to get complex now.
Nice one 😁😍
👏👏👏
😍😍😍
You sir just gained a new follower from this lol
Thanks
The equation you show is wrong,(-2(plusorminus)sqrt(4-9))/2 is equal to -1(plusorminus)i(sqrt(5)/2
Yeah you are right. It is my bad. Thanks for mentioning 💛
You just removed the square root of 5 magically. you can't do that. You can take out the negative since it's i but the square root of 5 has to stay
Oh that's my bad. Thanks for mentioning.
very well explained! thanks
Glad it was helpful!
This video has clearly explained the notations :)
Happy to hear that. I'll try to elaborate on each notion with examples in my next videos. Thanks for watching
Related to quantum mechanics.....do they rise question in 12th board cbsc
I'm afraid I don't know
sir, can u make more videos on quantum mechanics in 3D
Of course. I'll be solving more cases in 3D
the guy who committed to making free vst for every synthesis process rofl. i managed to fenangle amplitude and phase for circular membrane from falstad's physics applets in the old days. so i appreciate this video. i do need to make spherical bouncy sounds on my current project but i'll uuh i'll see if chat can cough up a list of coefficients thankyou :) a lot of educated people don't seem to use // init // s0 = 1; s1 = 0; // loop // s0 -= w * s1; s1 += w * s0; renormalise it every couple buffers.. well that's my scope
You are amazing, thanks for breaking it down because in griffiths it's a whole zoo!
Glad you liked it Leona. 🥰
8:55