Видео

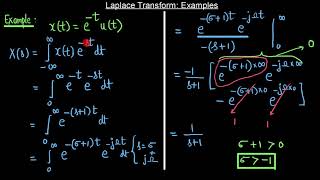
the great video i had a lot of confusions but finished in not more than 30 mins you're life saver

how we got the integration at 18:08 please and thank you

Isidro Burgs

in case 2 how did u get the t^2 ? it should have been taken outside the integral sign because you are integrating with respect to tau

At 20:20, your integration is incorrect. I did it many times and got the same answer, but different than yours. I also checked it with online calculators.

I'm sorry to say there are a lot of mistakes in the explanations and even in the equations you gave at the end the function y(t) exists only between 0 and 1.

When you reach the time period of {2 ≤ t ≤3} wouldn't the function in your integral be just 1? Since the area of convergence/overlapping occuring is just up to the amplitude (or just a square decreasing) of x(t) = 1?

Could one exolpalin to me why he didn't put 2 in equation of line is 2-t why in his integrations didn't put 2 ??

nice explanation

Bro I can actually understand you and hear you very clearly. Legend

Thanks a lot

Hey! Through a projection, you were able to demonstrate that Cos and Sin are two different components of eiθ. But Mathematically, we show that eiθ = cos θ + isinθ through Taylor's theorem, on the argument that both eiθ and cos θ + isin θ can be expressed into the same exponential. Since a direct visual demonstration of the identity can be established, isn't it possible to go directly from eiθ to cos θ + isin θ without showing that both can be converted to some same other thing?

so for homogeneity(scaling) we use the power of x and y?

Neso waale bhaiya ho kya aap

I think WRONG SOLVING AT 18:00

your voice is similar to neso academy signals and systems teacher

😍

Спасибоо❤😊

thank you.

Example 4 is function

Disappointing, too much playing about with the computer graphics.

U Neso Academy Guy??

The blue hatching is often wrong. It should run all the way up to the red response function h, wherever x is 1.

what is slope?

o nooo, how you find t- tawo

very nice explanation

amplitude of impulse is infinity , right ? how this converter works ?

According to the first condition if we suppose a function f=1/√x in for [0,2] with time period 2 will it have Fourier transform bcoz it is absolutely integrable but has infinite value at 0??

I want to ask, what software do you use in drawing this lecture?

if in the convolution I assume t=0, then how will you solve the integration?

thank you , it's so practical

really helpfull. lot of love

Please someone clearly tell me how we are getting (t-2)on the left hand side after time reversal, I can not get that. In my sense it would be (t+2) but. Please clearly tell someone shifting process after time reversal.

thank u!

Sir, what is difference between Verilog and VHDL? Which is better?

thankyou very much !!!!!

Y(t)=0 for t>3....I think

U explain in the best way i have read

Thank you for the explanation, good sir

ur tone of voice makes me want to break my headphones

Nice one sir🙏

Thank you for a very nice explanation. This is by far the best signal processing series I have seen on youtube! More people should watch this :)

Thank you ! very well explained

Brilliant explaination , sir

This is the example of dirichilet condition ?

32:00

Sorry but the concept of recovering a signal from its samples is an easy concept it doesn't need 18min it's too long you should instead make the computations using the fourier transform to explain how mathematically you can recover it ! Thanks for your effort anyway !!

Please note that the vertical axis in the last diagram x(-t) is at -1.

I have seen various videos in order to understand the topic .....got that into my mind after seeing the elaborate explanation in video......thankyou sir

Your handwriting and drawings , symbols such as Ω and x , 𝜏 are very attentive, speech is very clear. Thank you so much for your effort 👌🏻🙏🏻