Видео

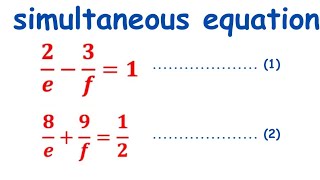
Thank you sir

Let say x=29 What is y ?It wouldn't be integer but.....y=1/(1/13-1/29)

aa + bb = 6100 *. 6100 = 3600 + 2500 = 60x60 + 50x50 ** a = 60, b= 50 *** a+ b = 60+50 = 110./.

thank you

The mean is wrong

Mean is=46.5

I appreciate your doing but the mean is wrong sir

❤

This topic used to killme in my brain in maths Thanks

Mean is wrong i got 46.5

Mean is very wronh Its 46.5

Thank you sir

🙏

thanks i was struggling with a project 💀

theres also 7 more negative solutions between -10 and 0

I love it ❤

-3,5^2-(-3,5^3)=30,6 <=> -3,672^2-(-3,672)^3=36,0 <=> x=-3,672

the process is too long. just take the square root and solve.

it doesn't have to be so complicated. 2 works too

I'm not watching more than 1 min, but substituting 9+27 for 36 seems like an ass-pull. If that's allowed, then just say x=-3 by inspection, then divide and solve the quadratic (by completing the square...using formula in an olympiad?!) to get x=2 plus or minus 2i root2. Done in a minute.

The video was very helpful thank you sir

-3. Take it or leave it.

3:13 - why not keeping with the nice (a-b)*(a+b) trick? [(x-4)^2 - x^2] * [(x-4)^2 + x^2] = 0 <=> (x-4)^2 - x^2 = 0 (1) OR (x-4)^2 + x^2 = 0 (2) (1) [(x-4) + x] * [(x-4) - x] = 0 (2*x - 4) * (-4) = 0 x = 2 (root 1) (2) in R: (x-4)^2 + x^2 = 0 <=> x-4 = 0 AND x = 0 => no roots in C: (x-4)^2 - (i*x)^2 = 0 [(x-4) - i*x] * [(x-4) + i*x] = 0 <=> (1-i)*x - 4 = 0 OR (1+i)*x - 4 = 0 <=> x = 4/(1-i) = [4*(1+i)]/[(1-i)*(1+i)] = 2 + 2*i (root 2) OR x = 4/(1+i) = [4*(1-i)]/[(1-i)*(1+i)] = 2 - 2*i (root 3)

Taking the 4th root, x-4= can't be +x, but could be -x . x=2 works. There may be complex no. solutions, as well. Check video. Yes, there are.

Pra que tudo isso se na terceira linha já tinha se encontrado a resposta?

Power both sides by 1/4, now it's middle school math

x=-2

Please interquartile deviation and mean deviation are the same or different

49=(V7)^4 , (V(24-8x))^2=(4x)^2 , 24-8x=16x^2 , /:8 , 3-x=2x^2 , 2x^2+x-3=0 , x=(-1+/-V(1+24))/4 , x=(-1+/-V25)/4 , x=(-1+/-5)/4 , x= 1 , -3/2 , test , x=1 , 49=49 , OK , x=-3/2 , 1/343=1/343 , OK ,

I definitely enjoyed the video

Thank You So Much Sir !

Beautiful video🖤👏🏾

God will bless you sir

i have watched a number of videos teaching his topic but this the most summarized and simplest video to understand. the explanation is just on point and the detailing of the meaning of each symbol in the formulas makes it easier for someone to understand better

Understood well❤🎉

Please tell me how you get the frequency please is very urgent

Tell me how did you get the "F"

Similar solution as that one of @GyB2GyB2GyB: transform to 2^3x - 2^x = 120 . Assume 2^X = a : a^3 - a = 120 or a(a^2 - 1 ) = 120 or a(a - 1 ) ( a + 1 ) = 2.2.2.3.5 = 5.4.6= 120 a=5 = 2^x x = log 5 base 2 = 2.3219 8^x = 125 8^x - 2^x = 125 - 5 = 120 Very fast solution with not much calculation needed. Calculator used for checking only.

Is it not important to first find the real class boundaries before finding the mean..like to close the gaps between the class limits??

y ^ 3 - y = 120 y * (y ^ 2 - 1) = 120 y * (y - 1) * (y + 1) = 2 * 2 * 2 * 3 * 5 (y - 1) * y * (y + 1) = (2*2) * 5 * (2*3) (y - 1) * y * (y + 1) = 4 * 5 * 6 (y - 1) * y * (y + 1) = (5 - 1) * 5 * (5 + 1) y = 5

Hello Chorimaths, there seems to bee an error in your solution method. Your x = 2024 yroot 2024 is effectively X ^ X. Please check your solution in the initial equation X ^X ^ 2024 = 2024 . Your X = 2024 yrootx 2024 or X = 2024 ^ 1/2024 will result in 2082,9 instead of 2024. X ^ X = 2024 yrootx 2024 = 1,0037683626, and X = 1,0037542417

Excellent! Thanks so much.

Where does it tell you that only real solutions are required?

I have simple way We know that, (A+B)^2=(A-B)^2+4AB Apply this formula 500=64+4AB 4AB=436 AB=109 Hope this will be useful 👍 From Chennai India

The error is the misuse of the square root property.

Mean is wrong

8^2,33-2^2,33=122 <=> 8^2,322 - 2^2,322= 120 <=> x=2,322

Whereas guess 'n' test is a legitimate technique, it is not very reliable, also, you failed to check y=4 without explanation. If we factorize, we get (y-1)y(y+1)=120 That is, 3 successive integers whose product is 120. these must be close to the cube root of 120, so we see 3, 4, 5 work. y = 5. The problem with guess 'n' test is that it can be a loong process. Further, you should have indicated WHY you expected an integer solution, you know: "Any cubic polynomial must have at least one real solution and if a monic polynomial with integer coefficients has real solutions they must be integers." You can't assume the student knows this stuff. Further, There is no indication that the solutions be real, so you MUST find the complex solutions too. (Complex, not imaginary - complex numbers have an imaginary PART). If you want only real solutions you have to say "real solutions". I mean, how hard is that?

100^200^24 10^10^10^20^24 2^52^52^5^5^4^4^6 1^1^1^1^1^1^1^2^2^2^2^3^2 1^1^1^1^3^2 (x ➖ 3x+2) .

120/8÷10.40x^Log^x120/2=60 {10.40 ➖ 60}= 10.20 10.5^4 2^5.5^4 2^1.1^4 2..2^2 1.1^2 1^2 (x ➖ 2x+1) .