
- Видео 209
- Просмотров 2 744 769
Science of Fluids
Ирландия
Добавлен 20 фев 2012
Dr. Wanan Sheng is a fluid dynamicist, with experience of more than 30 years in many areas in fluid dynamics, including hydrodynamics, aerodynamics and wave energy conversion. Recently research work on the fundamentals of fluid dynamics has shown the inconsistencies in fluid dynamic equations and for better understanding to fluid dynamics. This would include all aspects in fluid dynamics.
The talks on fluids aim for:
- introducing the fundamentals and principles for fluid dynamics;
- providing the better understandings for the basics of fluid dynamics;
- lecturing on the skills to use the mathematical tools to solve practical problems;
- lectures on different topics, including the fundamentals of fluid dynamics, potential flows, boundary element method, wave theory, boundary layer theory, turbulence models etc.
If you like my talks, please support me by subscribing my RUclips channel.
Thank for your interest and your support.
The talks on fluids aim for:
- introducing the fundamentals and principles for fluid dynamics;
- providing the better understandings for the basics of fluid dynamics;
- lecturing on the skills to use the mathematical tools to solve practical problems;
- lectures on different topics, including the fundamentals of fluid dynamics, potential flows, boundary element method, wave theory, boundary layer theory, turbulence models etc.
If you like my talks, please support me by subscribing my RUclips channel.
Thank for your interest and your support.
Basic Fluid Dynamics: Derivations of Bernoulli's equation, Part 3, The incompressible flows
Chanel: Science of Fluids, ruclips.net/user/ScienceofFluids
Playlist: Fluids made easy: 06- Fundamentals of potential flow theory
ruclips.net/p/PLoAFvHT_L-W8rm8Gg7ytsnWDEBLsBrQsW
This talk provides an introduction on why we need to derive the Bernoulli's equation for potential flows, and the derivation of the Bernoulli's equation for potential flows. Relatively, under the assumption of potential flows, the Bernoulli's equation can be more easily derived and in fact more useful in practical applications, since finding out the streamlines is not an easy task, even when the flow field is solved, while the Bernoulli's equation for potential flows is valid for the entire flow field, thus the appl...
Playlist: Fluids made easy: 06- Fundamentals of potential flow theory
ruclips.net/p/PLoAFvHT_L-W8rm8Gg7ytsnWDEBLsBrQsW
This talk provides an introduction on why we need to derive the Bernoulli's equation for potential flows, and the derivation of the Bernoulli's equation for potential flows. Relatively, under the assumption of potential flows, the Bernoulli's equation can be more easily derived and in fact more useful in practical applications, since finding out the streamlines is not an easy task, even when the flow field is solved, while the Bernoulli's equation for potential flows is valid for the entire flow field, thus the appl...
Просмотров: 654
Видео
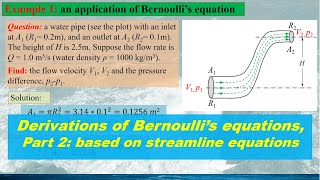
Derivations of Bernoulli's equation, Part 2: the derivation based on streamline equations
Просмотров 3502 года назад
Chanel: Science of Fluids, ruclips.net/user/ScienceofFluids Playlist: Fluids made easy: 06- Fundamentals of potential flow theory ruclips.net/p/PLoAFvHT_L-W8rm8Gg7ytsnWDEBLsBrQsW This talk provides an easy and more understandable way to derive the Bernoulli's equation, based on the simple streamline equations and the Euler equations. As such, the derivation of the Bernoulli's equation (the most...
Double model test in large waves at Plymouth wave tank
Просмотров 7942 года назад
Double model test in large waves at Plymouth wave tank
Small OctaOWC model tested in the wave tank
Просмотров 3372 года назад
Very large pitch motion, but the model does not capsize
Huge waves splash at the end of the tank
Просмотров 1,9 тыс.2 года назад
Huge waves splash at the end of the tank
Edinburgh University FloWave Wave Sprouts: The Triple, Double and Single Sprouts of Waves
Просмотров 9632 года назад
Significant wave generation at Edinburgh FloWave 360 deg Tank
Large wind-generated waves: good for surfing practice
Просмотров 992 года назад
surfing practice at Garrestown, South Cork
The Giant Causeway in Northern Ireland
Просмотров 582 года назад
The Giant Causeway in Northern Ireland
2.5-hour nature sound of a flowing water and birds chirpping
Просмотров 1002 года назад
2.5-hour nature sound of a flowing water and birds chirpping
WaveScan vs ODAS buoy: model test in large regular waves
Просмотров 3872 года назад
WaveScan buoy (left) vs ODAS buoy (Ocean Data Acquisition System) - 1:8 WaveScan model capsized while 1:16 WaveScan survived (left) - 1:8 ODAS buoy survived (the small model is 1:15 WaveRider model)
Waves coming to a narrow small beach
Просмотров 752 года назад
Waves in a shallow water are very flat at the troughs but sharp at the peaks...
Large wind-generated waves in the coast of Ireland (4)
Просмотров 742 года назад
Large wind-generated waves in the coast of Ireland (4)
How we can derive Bernoulli's equation, Part 1: the derivation based on direct physics
Просмотров 3702 года назад
How we can derive Bernoulli's equation, Part 1: the derivation based on direct physics
The streamlines and pathlines in unsteady flows: why they are so different?
Просмотров 8432 года назад
The streamlines and pathlines in unsteady flows: why they are so different?
The streamlines and pathlines in steady flows: why they are same?
Просмотров 8102 года назад
The streamlines and pathlines in steady flows: why they are same?
Why we are not using the Lagrangian Approach in fluid dynamics?
Просмотров 6922 года назад
Why we are not using the Lagrangian Approach in fluid dynamics?
Panel method for potential flows, Part 3: boundary integral equation for 2D flows
Просмотров 7773 года назад
Panel method for potential flows, Part 3: boundary integral equation for 2D flows
Potential flow theory for incompressible flows, Part 1: principles and equations
Просмотров 3073 года назад
Potential flow theory for incompressible flows, Part 1: principles and equations
Panel method for potential flows, Part 2: Boundary integral equations in 3D flows
Просмотров 1,4 тыс.3 года назад
Panel method for potential flows, Part 2: Boundary integral equations in 3D flows
Panel method for potential flows, Part 1: Green's theorem
Просмотров 1 тыс.3 года назад
Panel method for potential flows, Part 1: Green's theorem
Elementary potential flows, the simple and useful combinations for practical potential flows
Просмотров 9213 года назад
Elementary potential flows, the simple and useful combinations for practical potential flows
Elementary potential flows, the building stones for potential flow theory, Part 3, dipoles
Просмотров 2463 года назад
Elementary potential flows, the building stones for potential flow theory, Part 3, dipoles
Elementary potential flows, the building stones for potential flow theory, Part 2, sources and sinks
Просмотров 3203 года назад
Elementary potential flows, the building stones for potential flow theory, Part 2, sources and sinks
Elementary potential flows, the building stones for potential flow theory. Part 1: uniform flows
Просмотров 5133 года назад
Elementary potential flows, the building stones for potential flow theory. Part 1: uniform flows
An easy way to derive Bernoulli's equation by using the streamline equations and Euler equations
Просмотров 5883 года назад
An easy way to derive Bernoulli's equation by using the streamline equations and Euler equations








0:40 wow the double rainbow, i like that😍
What causes the arch?
Thanks for your interest. It is an assumption as the norm as ns equation. No particular reason for that.
hi, thank you for your video. can you please explain why at 31:04 we get the factor of 1/3 in front of the kinematic viscosity? it's not there in the equation above it. Thank you.
awesome
Thank you, sir.
شرح رائع شكرا.
perfect . it's help my lessons in university .
thank you for your comments and the interest in my talk
this proof is far better than cramming maclaurin or taylor
13:10 shouldn't we use different formula of τij = μ ∂uj/∂xi with the row vecror version of divergence formula? Then the answer would be correct
Yes, you are right about the definition, both ways are correct, but not mixed expressions. Thank you.
Cant believe there exist a place called "Cork"
Cork is the 2nd largest city in Ireland
@@ScienceofFluids is it where wine corks come from?
In fact, cork from Irish means marsh
@@ScienceofFluids y'all name things so funny 😂
this is really well done
thank you
Thank you for clearing this doubt... I was really confused earlier. This is the best video on the topic❤❤.
Thank you
What is the critical Reynolds number when the flow passes through an infinitely long object with a semicircular head? Let me ask what the critical Reynolds number is in this case. thank you
Many thanks for the fantastic video. I am not sure about this, but the equation for phi as a integral of greens function with certain strength at 18:49 may be wrong. Shouldn't it be phi(X0)=int(sigma(X0)*G(X;X0)) instead of phi(X)=?
I think it should be phi(X), the velocity potential in the field, because the integral is on the surfaces, that is, for different X0.
He made a double rainbow
0:42 double rainbow
ruclips.net/video/_-N2sovNYpA/видео.htmlsi=6C7Fx6X6tjm7k0P1&t=149 "In the frequency domain the pressure can be expressed as" - is this like a lecture? some justification? the omega of what? Sadly most of hydrodynamics have lots of assumptions not really justified or clarified.
Yes, it is a lecture. And yes, there are assumptions for the hydrodynamic forces, but it is a very useful and reliable method for getting the forces, and these can be used for industrial design. Or we can say the best method, but not the most complicated one.
@@ScienceofFluids I found your lectures since I have hydrodynamics course based on Newman book. Its hard go go through since almost any equations have so many assumptions and there are just few if any explanations. Some are obvious (like linearization terms) but rest not that much.
@@WojciechowskaAnna yes, that's because the full fluid dynamics is so complicated, the nonlinear 2nd-order partial differential equations, and so far there are no good methods to solve them. However, it is found that the assumptions, such as the potential flow theory, are good enough for many practical problems, as in aerodynamics and in hydrodynamics (wave-structure interactions). otherwise you have to use CFD for solving the problems, very very computational demanding. And also CFD is based on the turbulence models (again the assumptions). so this is the reality for fluid dynamics. you have to simplify the problem, or you can go nowhere.
@@ScienceofFluids i undurstand the need of simplification, I just wish that that there would be more explanations of "why some assumptions can be done". When I go through many boundary conditions - they are usually listed, with little explanation. I am studying it, so most of them are see first time - and I just wish books and lecturures would cover more this subject.
@@WojciechowskaAnna I have published a book in 2022, 'Fundamentals of Wave Energy Conversion', published by Eliva Press. The first few chapters are on potential flows, and I explained why we can use potential flows for our practical problems (I would say I didn't find such explanations in anywhere). Of course, I have talked about the boundary conditions in the book too. if you are interested, I can send you a chapter of the book on potential flows.
❤❤❤❤❤
Thank you
I want to sit in it in a rubber dinghy
Thanks a lot , really good content
Excellent work ❤
Thanks a lot 😊
pretty
Wow
Thank you so much for this.
I am not sure how much the machine costs, but I know it is manufactured by Edinburgh Design.
how much is this machine?
The wavemakers have 3m depth.
@@ScienceofFluids i mean money, how much is this. Thanks.
hi. thank you so much. it was really usefull for me.
Lame. Let me in the control cabine and I will produce a soliton wave with my special sequence 😉🌊💕you will have to clean up the roof afterwards 😜
I would like to see that, but unfortunately 0:44 we can't do that.
@@ScienceofFluids It's a shame it doesn't work. It's relatively simple you need to figure out the Resonance frequency of the full vet by doing a frequency swipe you watch for non lineare Effects then you modulate multiple frequencies into the vet such that the phase velocity exceeded the group velocity such that non linear effect add up. The waves will add up to a single huge wave and that is then the Soliton wave. Also if the depth of the vet decrease in the end like a beach then you will get a even more higher wave aka a tsunami.🌊😉😁
At 20:07, if the third trm goes to zero due to incompressible condition, then why did you include it again in your next slide? just like you omitted the incompressibility effect part from the second term, why didn't you directly omit the 3rd term completely?
In fluids, there are some cases the fluid compressibility must be included. that's why the compressibility term must be included in the general N-S equation. Thank you.
@@ScienceofFluids Oh, but then why didn't you include the part that you had omitted from the second term. Because even that part was omitted by applying the continuity equation for incompressible flow right?
How to calculate laplacian
If you watch my video 'Derivation of the Navier-Stokes equation', you can see how the Laplacian is calculated. Thank you.
Very educating video. I keep coming back to it to refresh my knowledge on this topic. However i have a question: what if the radiation potentials were to be solved for not just the rigid body modes but also include the flexible modes, how can these be done essentially with the change of the boundary condition? Thanks in advance
I recently saw some developments on the flexible wave energy converters, and I think you can find some useful information from that development. Google search would lead you there.
Someone realy just said "lets turn it on max power for a bit and see what happens."
It would be interesting, but it would be also damaging...
I'd love to swim that.
Thank you. it would be interesting in swimming in large waves...
Thank you very much, you helped me a lot with this video. Can you make a short comment from where the factor 1/3 in the last term stems from? (firstly seen at 30:55)
10:43 in the video in the compressibility part of the Stokes's matrix. Might have something to do with the -2/3 factor. My hunch is that is a simmilar assumption as seen with common derivations of pressure, regarding there are 3 directions (x,y,z), of which 1 does not leave the plane.
good job
In which book can I find these equations derived? Talking about minute 10:57?
I think in many books on fluid mechanics you can find the derivations. the books I would recommend are John Anderson's 'fundamentals of Aerodynamics', or Frank White's 'fluid mechanics'.
Could businesses create rainbows to attract customers?
like a water fountain?
Nice video. It teaches more each time one goes over it. But I'd like to ask,how can it be used to remove irregular frequency effects?
Thank you for clear illustration!
0:42 Not even my rainbows come out that sharp
what's dx+?
It is the grid number in streamwise direction.
Amazing!
Thank you very much
Thank you and I have a doubt about the Divergence of tensor, It is mentioned in different ways in different books. ∇ · (φT) = φ∇ · T + T ^⊤ . ∇φ ---- eq(1) and ∇ · (φT) = φ∇ · T + T ∇φ ---eq(2). Which one is correct, if T is tensor. Then how to solve this one ∇ · τ = ∇ · (2µE), Here E is strain tensor
Thanks for asking. These may be both correct, but depending on how the tensor component is defined. for instance, Tij can be defined as a component in i-direction on a plane of constant y, or in j-direction on a plane of constant x. these two different definitions would cause different expressions as you have seen in different books. Hope this helps.
@@ScienceofFluids Thanks a lot. Explanation is really helpful
Greetings prof.thankd alot for these videos.please what changes when we consider forward speed and zero speed?
Prof this is beautiful. I'd like to ask,how do I determine the size of the matrices? Secondly while evaluating the green function, what transformation occurs between the body panel and the source point? Thank you in advance
the matrix size is dependent on how many motion modes are included. for a single body, there should be 6 DOFs, thus the matrix is 6*6. and for a 2-body system, there should be 12 DOFs, thus the size of matrix is 12*12. I don't know what is the second question, can you specify it more?
@@ScienceofFluids thank you prof. The second question is this: is there any transformation of the body mesh or panels to in order to get the radiation potentials in a 3D case? What i mean is how do we get the parameters for the green function inputs;r and eta as in G(r,eta)? Thanks
I see, but I have no idea how the transformation can be done. However, there are open source software, and you may not need to go for such detailed calculation. I wonder whether you can find something in HAMS (Hydrodynamic Analysis of Marine Structure) and the relevant publications.
@@ScienceofFluids thank you very much prof. I will check hams then
Thank you professor. There is a suggestion to add references of articles, journals or books in the description.
Thanks for suggestions. I will see to add some more information in the description.
The news of the queens death had them out with sprinklers
bro imagine being in there